Jump to content

File:Wakes near the period 1 continent in the Mandelbrot set.png

Page contents not supported in other languages.
This is a file from the Wikimedia Commons
From Wikibooks, open books for an open world

Original file (4,096 × 4,096 pixels, file size: 1.54 MB, MIME type: image/png)

Summary

Description
English: Wakes near the period 1 continent in the Mandelbrot set. Boundary of the Mandelbrot set rendered with distance estimation (exterior and interior). Labelled with periods (blue), internal angles and rays (green) and external angles and rays (red).
Date
Source Own work
Author Claude Heiland-Allen
Other versions
Wakes along the main antenna in the Mandelbrot set
Wakes near the period 3 island in the Mandelbrot set

Summary

This image is made with c code, see : http://code.mathr.co.uk/mandelbrot-graphics/blob/HEAD:/c/bin/m-subwake-diagram-a.c

Dependencies :

C src code

/*
http://code.mathr.co.uk/mandelbrot-graphics/blob/HEAD:/c/bin/m-subwake-diagram-a.c
by Claude Heiland-Allen
*/

#include <mandelbrot-graphics.h>
#include <mandelbrot-numerics.h>
#include <mandelbrot-symbolics.h>
#include <cairo.h>

const double twopi = 6.283185307179586;

void draw_label(m_image *image, m_d_transform *transform, double _Complex c0, const char *text, double pt, m_pixel_t colour) {
  double _Complex c = c0;
  double _Complex dc = 1;
  m_d_transform_reverse(transform, &c, &dc);
  cairo_surface_t *surface = m_image_surface(image);
  cairo_t *cr = cairo_create(surface);
  cairo_select_font_face(cr, "LMSans10", CAIRO_FONT_SLANT_NORMAL, CAIRO_FONT_WEIGHT_NORMAL);
  cairo_set_font_size(cr, pt);
  cairo_text_extents_t te;
  cairo_text_extents(cr, text, &te);
  cairo_move_to(cr, creal(c) - te.x_bearing - te.width / 2, cimag(c) - te.y_bearing - te.height / 2);
  cairo_text_path(cr, text);
  cairo_set_source_rgba(cr, m_pixel_red(colour), m_pixel_green(colour), m_pixel_blue(colour), m_pixel_alpha(colour));
  cairo_fill(cr);
  cairo_destroy(cr);
}

void draw_internal_ray(m_image *image, m_d_transform *transform, int period, double _Complex nucleus, const char *angle, double pt, m_pixel_t colour) {
  int steps = 128;
  mpq_t theta;
  mpq_init(theta);
  mpq_set_str(theta, angle, 10);
  mpq_canonicalize(theta);
  double a = twopi * mpq_get_d(theta);
  mpq_clear(theta);
  double _Complex interior = cos(a) + I * sin(a);

  double _Complex cl = 0, cl2 = 0;
  double _Complex c = nucleus;
  double _Complex z = c;
  cairo_surface_t *surface = m_image_surface(image);
  cairo_t *cr = cairo_create(surface);
  cairo_set_source_rgba(cr, m_pixel_red(colour), m_pixel_green(colour), m_pixel_blue(colour), m_pixel_alpha(colour));
  for (int i = 0; i < steps; ++i) {
    if (2 * i == steps) {
      cl = c;
    }
    if (2 * i == steps + 2) {
      cl2 = c;
    }
    double radius = (i + 0.5) / steps;
    m_d_interior(&z, &c, z, c, radius * interior, period, 64);
    double _Complex pc = c;
    double _Complex pdc = 1;
    m_d_transform_reverse(transform, &pc, &pdc);
    if (i == 0) {
      cairo_move_to(cr, creal(pc), cimag(pc));
    } else {
      cairo_line_to(cr, creal(pc), cimag(pc));
    }
  }
  cairo_stroke(cr);
  if (a != 0) {
    double t = carg(cl2 - cl);
    cairo_save(cr);
    double _Complex dcl = 1;
    m_d_transform_reverse(transform, &cl, &dcl);
    cairo_translate(cr, creal(cl), cimag(cl));
    cairo_rotate(cr, -t);
    cairo_translate(cr, 0, -pt/3);
    cairo_select_font_face(cr, "LMSans10", CAIRO_FONT_SLANT_NORMAL, CAIRO_FONT_WEIGHT_NORMAL);
    cairo_set_font_size(cr, pt);
    cairo_text_path(cr, angle);
    cairo_fill(cr);
    cairo_restore(cr);
  }
  cairo_destroy(cr);
}

void draw_external_ray(m_image *image, m_d_transform *transform, const char *angle, m_pixel_t colour, double dx, double dy) {
  int maxiters = 1024;
  double r = sqrt(2);

  m_binangle btheta;
  m_binangle_init(&btheta);
  m_binangle_from_string(&btheta, angle);
  mpq_t qtheta;
  mpq_init(qtheta);
  m_binangle_to_rational(qtheta, &btheta);
  m_binangle_clear(&btheta);
  m_d_exray_in *ray = m_d_exray_in_new(qtheta, 8);
  mpq_clear(qtheta);

  cairo_surface_t *surface = m_image_surface(image);
  cairo_t *cr = cairo_create(surface);
  cairo_set_source_rgba(cr, m_pixel_red(colour), m_pixel_green(colour), m_pixel_blue(colour), m_pixel_alpha(colour));
  bool first = true;
  for (int i = 0; i < maxiters; ++i) {
    if (m_failed == m_d_exray_in_step(ray, 64)) {
      break;
    }
    double _Complex c = m_d_exray_in_get(ray);
    if (cabs(c + 0.75) > r) {
      continue;
    }
    double t = carg(c + 0.75);
    double _Complex dc = 1;
    m_d_transform_reverse(transform, &c, &dc);
    if (first) {
      cairo_save(cr);
      cairo_translate(cr, creal(c) + dx, cimag(c) + dy);
      cairo_rotate(cr, -t);
      cairo_select_font_face(cr, "LMMono10", CAIRO_FONT_SLANT_NORMAL, CAIRO_FONT_WEIGHT_NORMAL);
      cairo_set_font_size(cr, 48);
      cairo_text_path(cr, angle);
      cairo_fill(cr);
      cairo_restore(cr);
      cairo_move_to(cr, creal(c) + dx, cimag(c) + dy);
      first = false;
    } else {
      cairo_line_to(cr, creal(c), cimag(c));
    }
  }
  cairo_stroke(cr);
  cairo_destroy(cr);
}

int main(int argc, char **argv) {
  (void) argc;
  (void) argv;
  int w = 4096;
  int h = 4096;
  complex double c = -0.75;
  double r = 1.75;
  double er = 600;
  int maxiters = 8192;
  const char *filename = "subwake-diagram-a.png";

  m_pixel_t red   = m_pixel_rgba(1, 0, 0, 1);
  m_pixel_t green = m_pixel_rgba(0, 0.5, 0, 1);
  m_pixel_t blue  = m_pixel_rgba(0, 0, 1, 1);
  m_pixel_t black = m_pixel_rgba(0, 0, 0, 1);
  m_pixel_t white = m_pixel_rgba(1, 1, 1, 1);

  int retval = 1;
  m_image *image = m_image_new(w, h);
  if (image) {
    m_d_transform *transform = m_d_transform_rectangular(w, h, c, r);
    if (transform) {
      m_d_colour_t *colour = m_d_colour_minimal(white, black, white);
      if (colour) {
        m_d_render_scanline(image, transform, er, maxiters, colour);

        double _Complex c3, c4a, c4b, c5, c3c2, c2c3;
        m_d_nucleus(&c3, 0 + I * 1, 3, 64);
        m_d_nucleus(&c4a, 0.25 + 0.5 * I, 4, 64);
        m_d_nucleus(&c4b, 0.25 - 0.5 * I, 4, 64);
        m_d_nucleus(&c5,  0.3 + 0.3 * I, 5, 64);
        m_d_nucleus(&c3c2, c3 + I * 0.1, 6, 64);
        m_d_nucleus(&c2c3, -1 - 0.25 + 0.25 * I, 6, 64);

        double pt = 48;
        
        draw_internal_ray(image, transform, 1, 0, "1/2", pt, green);
        draw_internal_ray(image, transform, 1, 0, "1/3", pt, green);
        draw_internal_ray(image, transform, 1, 0, "1/4", pt, green);
        draw_internal_ray(image, transform, 1, 0, "1/5", pt, green);
        draw_internal_ray(image, transform, 1, 0, "3/4", pt, green);
        draw_internal_ray(image, transform, 2, -1, "0/1", pt, green);
        draw_internal_ray(image, transform, 2, -1, "1/3", pt, green);
        draw_internal_ray(image, transform, 3, c3, "0/1", 0.7 * pt, green);
        draw_internal_ray(image, transform, 3, c3, "1/2", 0.7 * pt, green);
        draw_internal_ray(image, transform, 3, c3, "1/3", 0.7 * pt, green);
        draw_internal_ray(image, transform, 3, c3, "1/4", 0.7 * pt, green);
        draw_internal_ray(image, transform, 3, c3, "3/4", 0.7 * pt, green);

        draw_external_ray(image, transform, ".(01)", red, 0, 0);
        draw_external_ray(image, transform, ".(10)", red, 0, 0);
        draw_external_ray(image, transform, ".(001)", red, 32, 32);
        draw_external_ray(image, transform, ".(010)", red, -48, 0);
        draw_external_ray(image, transform, ".(011)", red, 0, 0);
        draw_external_ray(image, transform, ".(100)", red, 0, 0);
        draw_external_ray(image, transform, ".(0001)", red, 0, -16);
        draw_external_ray(image, transform, ".(0010)", red, 16, 16);
        draw_external_ray(image, transform, ".(1101)", red, 0, 0);
        draw_external_ray(image, transform, ".(1110)", red, 0, 0);
        draw_external_ray(image, transform, ".(00001)", red, 0, 0);
        draw_external_ray(image, transform, ".(00010)", red, 0, 16);
        draw_external_ray(image, transform, ".(001010)", red, -32, -32);
        draw_external_ray(image, transform, ".(010001)", red, 0, 0);
        draw_external_ray(image, transform, ".(010110)", red, 0, 0);
        draw_external_ray(image, transform, ".(011001)", red, 0, 0);
        draw_external_ray(image, transform, ".(001001010)", red, -48, -48);
        draw_external_ray(image, transform, ".(001010001)", red, 0, 0);
        draw_external_ray(image, transform, ".(001001001010)", red, 0, 0);
        draw_external_ray(image, transform, ".(001001010001)", red, -16, -16);
        draw_external_ray(image, transform, ".(010010001010)", red, 32, 0);
        draw_external_ray(image, transform, ".(010010010001)", red, 0, 0);

        draw_label(image, transform,  0,   "1", 6 * pt, blue);
        draw_label(image, transform, -1,   "2", 3 * pt, blue);
        draw_label(image, transform, c3,   "3", 2 * pt, blue);
        draw_label(image, transform, c4a,  "4", 1.5 * pt, blue);
        draw_label(image, transform, c4b,  "4", 1.5 * pt, blue);
        draw_label(image, transform, c5,   "5", pt, blue);
        draw_label(image, transform, c2c3, "6", pt, blue);
        draw_label(image, transform, c3c2, "6", pt, blue);

        m_image_save_png(image, filename);
        retval = 0;
        m_d_colour_delete(colour);
      }
      m_d_transform_delete(transform);
    }
    m_image_delete(image);
  }
  return retval;
}

Licensing

I, the copyright holder of this work, hereby publish it under the following license:
w:en:Creative Commons
attribution share alike
This file is licensed under the Creative Commons Attribution-Share Alike 4.0 International license.
You are free:
  • to share – to copy, distribute and transmit the work
  • to remix – to adapt the work
Under the following conditions:
  • attribution – You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
  • share alike – If you remix, transform, or build upon the material, you must distribute your contributions under the same or compatible license as the original.

Captions

Add a one-line explanation of what this file represents

Items portrayed in this file

depicts

inception

17 February 2016

media type

image/png

File history

Click on a date/time to view the file as it appeared at that time.

Date/TimeThumbnailDimensionsUserComment
current14:21, 17 February 2016Thumbnail for version as of 14:21, 17 February 2016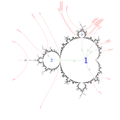4,096 × 4,096 (1.54 MB)CMUser created page with UploadWizard

Global file usage

The following other wikis use this file:

Retrieved from "https://en.wikibooks.org/wiki/File:Wakes_near_the_period_1_continent_in_the_Mandelbrot_set.png"