From Wikibooks, open books for an open world
Student’s t
Probability density function
Cumulative distribution function
Parameters
ν > 0 degrees of freedom (real )
Support
x ∈ (−∞; +∞)
PDF
Γ
(
ν
+
1
2
)
ν
π
Γ
(
ν
2
)
(
1
+
x
2
ν
)
−
ν
+
1
2
{\displaystyle \textstyle {\frac {\Gamma \left({\frac {\nu +1}{2}}\right)}{{\sqrt {\nu \pi }}\,\Gamma \left({\frac {\nu }{2}}\right)}}\left(1+{\frac {x^{2}}{\nu }}\right)^{-{\frac {\nu +1}{2}}}\!}
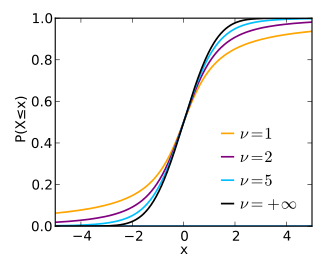
CDF
1
2
+
x
Γ
(
ν
+
1
2
)
⋅
2
F
1
(
1
2
,
ν
+
1
2
;
3
2
;
−
x
2
ν
)
π
ν
Γ
(
ν
2
)
{\displaystyle {\begin{matrix}{\frac {1}{2}}+x\Gamma \left({\frac {\nu +1}{2}}\right)\cdot \\[0.5em]{\frac {\,_{2}F_{1}\left({\frac {1}{2}},{\frac {\nu +1}{2}};{\frac {3}{2}};-{\frac {x^{2}}{\nu }}\right)}{{\sqrt {\pi \nu }}\,\Gamma \left({\frac {\nu }{2}}\right)}}\end{matrix}}}
2 F 1 is the hypergeometric function
Mean
0 for ν > 1, otherwise undefined
Median
0
Mode
0
Variance
ν
ν
−
2
{\displaystyle \textstyle {\frac {\nu }{\nu -2}}}
undefined
Skewness
0 for ν > 3, otherwise undefined
Ex. kurtosis
6
ν
−
4
{\displaystyle \textstyle {\frac {6}{\nu -4}}}
undefined
Entropy
...
MGF
undefined
CF
K
ν
/
2
(
ν
|
t
|
)
(
ν
|
t
|
)
ν
/
2
Γ
(
ν
/
2
)
2
ν
/
2
−
1
{\displaystyle \textstyle {\frac {K_{\nu /2}\left({\sqrt {\nu }}|t|)({\sqrt {\nu }}|t|\right)^{\nu /2}}{\Gamma (\nu /2)2^{\nu /2-1}}}}
Student t-distribution (or just t-distribution for short) is derived from the chi-square and normal distributions. We divide the standard normally distributed value of one variable over the root of a chi-square value over its r degrees of freedom. Mathematically, this appears as:
t
=
Z
χ
r
2
/
r
{\displaystyle t={\frac {\mbox{Z}}{\sqrt {\chi _{r}^{2}/r}}}}
where
Z
=
X
−
X
¯
σ
{\displaystyle Z={\frac {X-{\bar {X}}}{\sigma }}}
χ
r
2
=
χ
r
1
2
+
.
.
.
+
χ
r
n
2
{\displaystyle \chi _{r}^{2}=\chi _{r_{1}}^{2}+...+\chi _{r_{n}}^{2}}
↑ Hurst, Simon, The Characteristic Function of the Student-t Distribution , Financial Mathematics Research Report No. FMRR006-95, Statistics Research Report No. SRR044-95



![{\displaystyle {\begin{matrix}{\frac {1}{2}}+x\Gamma \left({\frac {\nu +1}{2}}\right)\cdot \\[0.5em]{\frac {\,_{2}F_{1}\left({\frac {1}{2}},{\frac {\nu +1}{2}};{\frac {3}{2}};-{\frac {x^{2}}{\nu }}\right)}{{\sqrt {\pi \nu }}\,\Gamma \left({\frac {\nu }{2}}\right)}}\end{matrix}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/02732e546784af1fb16d0dc1bb65dd743e2284ad)






