Trigonometry/Similar, Congruent, Isosceles, and Equilateral

At this point we're assuming:

• You already know what a triangle is
• You already know how to measure angles

We assume that you know that two triangles of different sizes can have exactly the same sized angles as each other.

One reason we assume you already know how to measure angles is that, actually, it is rather difficult to be mathematically precise about what we mean. What does it mean for one angle to be one third or twice another? A mathematically precise approach that we'll come to much later on, in book 2, is to divide angles repeatedly in half, and then build up larger angles from the smaller ones. For now we don't need to worry about this; it's enough to know how to measure angles, and to know some angles like $90^{\circ }$ and $45^{\circ }$.

About Exercises

The exercises in this book are here for your benefit. They are here to help you check for yourself that you are understanding what the book is saying.

Terminology

You need to know the meaning of these words for the next part

Isosceles triangle	Equilateral triangle

Terminology: Isosceles

• An isosceles triangle is a triangle with at least two sides that are the same length.

The picture of the isosceles triangle shows a small mark on the two sides that are the same.

When a triangle has two sides that are the same length, it is symmetric. Since it is symmetric, in our isosceles triangle the right and left sides are the same; it also has two angles that are the same.

Exercise: A quadrilateral with four different angles... If you have a quadrilateral (that is, a polygon with four sides) and two of them are the same length it does not have to be a symmetric quadrilateral. In a four sided polygon all the angles can be different even though two sides have the same length. Your task in this exercise is to draw a quadrilateral which has two sides the same length but that has all the angles different.

Testing whether a triangles is isosceles A triangle must be an isosceles triangle if either of the following is true: Some two sides are the same length. Some two angles are the same. If one is true, the other is automatically true too.

Isosceles triangle	Equilateral triangle

Terminology: Equilateral

• An equilateral triangle is a triangle with all three sides the same length.

An equilateral triangle happens also to be an isosceles triangle. If all three sides are the same length then we can certainly find two sides that are the same length! If that seems strange, well yes, it is different to other terminology we have for shapes. 'Triangle', 'square', 'pentagon', 'hexagon' are all different things. But an isosceles triangle can be an equilateral triangle, and it will be if all three sides are the same length.

Testing whether a triangle is equilateral A triangle must be an equilateral triangle if either of the following is true: All three sides are the same length. All three angles are the same. If one is true, the other is automatically true too.

Terminology: Similar and Congruent

Similar Triangles

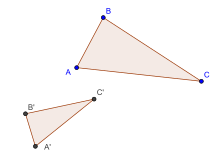
• Two triangles are congruent if they have the same shape and size. The diagram above does not show congruent triangles, because although the two triangles have the same angles the triangles are different sizes. The triangles in that picture are similar triangles.
  • If you can move, enlarge evenly or shrink evenly or reflect one triangle exactly onto another, or match one with another by doing a series of such steps, then they are similar.
  • If you can move or reflect one triangle exactly onto another, or match one with another by doing a series of such steps, then they are congruent.

Testing whether two triangles are congruent When two triangles are congruent, the angles of one triangle are the same as the angles of the other and the lengths of the sides of one triangle are the same as the lengths of the sides of the other. When we want to prove that two triangles are congruent, we don't need to prove all six correspondences. That's because, for example, once you know the lengths of the three sides of a triangle the angles are completely determined. There is only one triangle that can be made with sides of length 6 cm, 7cm and 8 cm. In the following we use 'S' as an abbreviation for Side, and A as an abbreviation for Angle, so (Side-Side-Side) is written as (SSS). There are four tests that can be used to prove that two triangles are congruent: The sides of one are all equal in length to the sides of the other (SSS). Two of the sides of one and the angle between those two sides equal the corresponding sides and angle of the other (SAS). Two of the angles of one and the side between those two angles equal the corresponding angles and side of the other (ASA). Both of the triangles are right angled, and the hypotenuse and another side of one are the same length as the hypotenuse and another side of the other.

There is only one kind of equilateral triangle, though they come in different sizes, so all equilateral triangles are similar to each other.

There are many different kinds of isosceles triangle. They aren't necessarily similar to each other.

Exercises

what do you mean by congruent and similar triangles? Give examples also. give at least 10 examples of each of them.

draw an equilateral triangle and measure its angles. Make some other ones too. Ans. 60

True or false: "All equilateral triangles are congruent to each other". Justify your answer .

Examples

Some examples of triangles are shown below, also showing the size of the angles.

Equilateral Triangle	45-45-90 Triangle	30-60-90 Triangle	50-60-70 Triangle	20-40-120 Triangle

Exercise: Terminology Two of the triangles above are isosceles. Which two? One of the triangles above has an obtuse angle (an angle greater than 90 degrees). Which one? Are any of the five triangles congruent to each other?