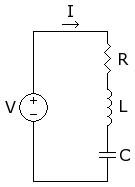
L
d
I
d
t
+
I
R
+
1
C
∫
I
d
t
=
V
{\displaystyle L{\frac {dI}{dt}}+IR+{\frac {1}{C}}\int Idt=V}
d
2
I
d
t
2
+
R
L
d
I
d
t
+
I
L
C
=
0
{\displaystyle {\frac {d^{2}I}{dt^{2}}}+{\frac {R}{L}}{\frac {dI}{dt}}+{\frac {I}{LC}}=0}
The characteristic equation is
s
2
+
R
L
s
+
1
L
C
=
0
{\displaystyle s^{2}+{\frac {R}{L}}s+{\frac {1}{LC}}=0}
s
=
−
α
±
α
2
−
β
2
{\displaystyle s=-\alpha \pm {\sqrt {\alpha ^{2}-\beta ^{2}}}}
Where
α
=
R
2
L
{\displaystyle \alpha ={\frac {R}{2L}}}
β
=
1
L
C
{\displaystyle \beta ={\frac {1}{\sqrt {LC}}}}
When
α
2
−
β
2
=
0
{\displaystyle {\sqrt {\alpha ^{2}-\beta ^{2}}}=0}
α
2
=
β
2
;
R
=
2
L
C
{\displaystyle \alpha ^{2}=\beta ^{2};R=2{\sqrt {\frac {L}{C}}}}
The equation only has one real root .
s
=
−
α
=
−
R
2
L
{\displaystyle s=-\alpha =-{\frac {R}{2L}}}
The solution for
I
(
t
)
=
A
e
−
R
2
L
t
{\displaystyle I(t)=Ae^{-{\frac {R}{2L}}t}}
The I - t curve would look like When
α
2
−
β
2
>
0
{\displaystyle {\sqrt {\alpha ^{2}-\beta ^{2}}}>0}
α
2
>
β
2
;
R
>
L
C
{\displaystyle \alpha ^{2}>\beta ^{2};R>{\frac {L}{C}}}
The equation has two real root .
s
=
−
α
±
α
2
−
β
2
{\displaystyle s=-\alpha \pm {\sqrt {\alpha ^{2}-\beta ^{2}}}}
The solution for
I
(
t
)
=
e
(
−
α
+
α
2
−
β
2
)
t
+
e
(
−
α
−
α
2
−
β
2
)
t
=
e
−
α
[
e
α
2
−
β
2
+
e
−
α
2
−
β
2
]
{\displaystyle I(t)=e^{\left(-\alpha +{\sqrt {\alpha ^{2}-\beta ^{2}}}\right)t}+e^{\left(-\alpha -{\sqrt {\alpha ^{2}-\beta ^{2}}}\right)t}=e^{-\alpha }\left[e^{\sqrt {\alpha ^{2}-\beta ^{2}}}+e^{-{\sqrt {\alpha ^{2}-\beta ^{2}}}}\right]}
The I - t curve would look like When
α
2
−
β
2
<
0
{\displaystyle {\sqrt {\alpha ^{2}-\beta ^{2}}}<0}
α
2
<
β
2
;
R
<
L
C
{\displaystyle \alpha ^{2}<\beta ^{2};R<{\frac {L}{C}}}
The equation has two complex root .
s
=
−
α
±
j
β
2
−
α
2
{\displaystyle s=-\alpha \pm j{\sqrt {\beta ^{2}-\alpha ^{2}}}}
The solution for
I
(
t
)
=
e
(
−
α
+
β
2
−
α
2
t
)
+
e
(
−
α
−
β
2
−
α
2
t
)
=
e
−
α
[
e
j
(
β
2
−
α
2
t
)
+
e
−
j
(
β
2
−
α
2
t
)
]
{\displaystyle I(t)=e^{\left(-\alpha +{\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}+e^{\left(-\alpha -{\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}=e^{-\alpha }\left[e^{j\left({\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}+e^{-j\left({\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}\right]}
The I - t curve would look like The damping factor is the amount by which the oscillations of a circuit gradually decrease over time. We define the damping ratio to be:
Circuit Type
Series RLC
Parallel RLC
Damping Factor
ζ
=
R
2
C
L
{\displaystyle \zeta ={R \over 2}{\sqrt {C \over L}}}
ζ
=
1
2
R
L
C
{\displaystyle \zeta ={1 \over 2R}{\sqrt {L \over C}}}
Resonance Frequency
ω
o
=
1
L
C
{\displaystyle \omega _{o}={1 \over {\sqrt {LC}}}}
ω
o
=
1
L
C
{\displaystyle \omega _{o}={1 \over {\sqrt {LC}}}}
Compare The Damping factor with The Resonance Frequency give rise to different types of circuits: Overdamped , Underdamped , and Critically Damped .
Δ
ω
=
2
α
{\displaystyle \Delta \omega =2\alpha }
For series RLC circuit:
Δ
ω
=
2
α
=
R
L
{\displaystyle \Delta \omega =2\alpha ={R \over L}}
For Parallel RLC circuit:
Δ
ω
=
2
α
=
1
R
C
{\displaystyle \Delta \omega =2\alpha ={1 \over RC}}
Q
=
ω
o
Δ
ω
=
ω
o
2
α
{\displaystyle Q={\omega _{o} \over \Delta \omega }={\omega _{o} \over 2\alpha }}
For Series RLC circuit:
Q
=
ω
o
Δ
ω
=
ω
o
2
α
=
L
R
L
C
=
1
R
L
C
{\displaystyle Q={\omega _{o} \over \Delta \omega }={\omega _{o} \over 2\alpha }={L \over R{\sqrt {LC}}}={1 \over R}{\sqrt {L \over C}}}
For Parallel RLC circuit:
Q
=
ω
o
Δ
ω
=
ω
o
2
α
=
R
C
L
C
=
R
C
L
{\displaystyle Q={\omega _{o} \over \Delta \omega }={\omega _{o} \over 2\alpha }={RC \over {\sqrt {LC}}}={R}{\sqrt {C \over L}}}
Because inductors and capacitors act differently to different inputs, there is some potential for the circuit response to approach infinity when subjected to certain types and amplitudes of inputs. When the output of a circuit approaches infinity, the circuit is said to be unstable . Unstable circuits can actually be dangerous, as unstable elements overheat, and potentially rupture.
A circuit is considered to be stable when a "well-behaved" input produces a "well-behaved" output response. We use the term "Well-Behaved" differently for each application, but generally, we mean "Well-Behaved" to mean a finite and controllable quantity.
When R = 0 , the circuit reduces to a series LC circuit. When the circuit is in resonance, the circuit will vibrate at the resonant frequency.
Z
L
=
Z
C
{\displaystyle Z_{L}=Z_{C}}
ω
L
=
1
ω
C
{\displaystyle \omega L={\frac {1}{\omega C}}}
ω
=
1
L
C
{\displaystyle \omega ={\frac {1}{\sqrt {LC}}}}
f
=
1
2
π
1
L
C
{\displaystyle f={\frac {1}{2\pi }}{\frac {1}{\sqrt {LC}}}}
The circuit vibrates and may produce a standing wave, depending on the frequency of the driver, the wavelength of the oscillating wave and the geometry of the circuit.
When R ≠ 0 and the circuit operates in resonance .
The frequency dependent components L , C cancel out ie ZL - ZC = 0 so that the total impedance of the circuit is
Z
R
+
Z
L
+
Z
C
=
R
+
[
Z
L
−
Z
C
]
=
R
+
0
=
R
{\displaystyle Z_{R}+Z_{L}+Z_{C}=R+[Z_{L}-Z_{C}]=R+0=R}
The current of the circuit is
I
=
V
R
{\displaystyle I={\frac {V}{R}}}
The Operating Frequency is
ω
=
1
L
C
{\displaystyle \omega ={\frac {1}{\sqrt {LC}}}}
If the current is halved by doubling the value of resistance then
I
=
V
2
R
{\displaystyle I={\frac {V}{2R}}}
Circuit will be stable over the range of frequencies from
ω
1
−
ω
2
{\displaystyle \omega _{1}-\omega _{2}}
The circuit has the capability to select bandwidth where the circuit is stable . Therefore, it is best suited for Tuned Resonance Select Bandwidth Filter
Once using L or C to tune circuit into resonance at resonance frequency
f
=
1
2
π
1
L
C
{\displaystyle f={\frac {1}{2\pi }}{\frac {1}{\sqrt {LC}}}}
The current is at its maximum value
I
=
V
R
{\displaystyle I={\frac {V}{R}}}
I
=
V
2
R
{\displaystyle I={\frac {V}{2R}}}
ω
1
−
ω
2
{\displaystyle \omega _{1}-\omega _{2}}
I
=
V
2
R
{\displaystyle I={\frac {V}{2R}}}
ω
1
−
ω
2
{\displaystyle \omega _{1}-\omega _{2}}
Circuit
General
Series RLC
Parallel RLC
Circuit
Impedance
Z
Z
=
(
j
ω
)
2
+
(
j
ω
)
R
L
+
1
L
C
{\displaystyle Z=(j\omega )^{2}+(j\omega ){\frac {R}{L}}+{\frac {1}{LC}}}
Z
=
1
R
L
C
1
(
j
ω
)
2
+
j
ω
1
R
C
+
1
L
C
{\displaystyle Z={\frac {1}{RLC}}{\frac {1}{(j\omega )^{2}+j\omega {\frac {1}{RC}}+{\frac {1}{LC}}}}}
Roots
λ
λ =
−
α
±
α
2
−
ω
o
2
{\displaystyle -\alpha \pm {\sqrt {\alpha ^{2}-\omega _{o}^{2}}}}
λ =
−
α
±
α
2
−
ω
o
2
{\displaystyle -\alpha \pm {\sqrt {\alpha ^{2}-\omega _{o}^{2}}}}
I(t)
Aeλ1 t + Beλ2 t
Aeλ1 t + Beλ2 t
Aeλ1 t + Beλ2 t
Damping Factor
α
{\displaystyle \alpha }
α
=
R
2
L
{\displaystyle \alpha ={R \over 2L}}
α
=
1
2
R
C
{\displaystyle \alpha ={1 \over 2RC}}
Resonant Frequency
ω
o
{\displaystyle \omega _{o}}
ω
o
=
1
L
C
{\displaystyle \omega _{o}={1 \over {\sqrt {LC}}}}
ω
o
=
1
L
C
{\displaystyle \omega _{o}={1 \over {\sqrt {LC}}}}
Band Width
Δ
ω
=
2
α
{\displaystyle \Delta \omega =2\alpha }
R
L
{\displaystyle {R \over L}}
1
C
R
{\displaystyle {1 \over CR}}
Quality factor
Q
=
ω
o
Δ
ω
=
ω
o
2
α
{\displaystyle Q={\omega _{o} \over \Delta \omega }={\omega _{o} \over 2\alpha }}
Q
=
L
R
L
C
=
1
R
L
C
{\displaystyle Q={L \over R{\sqrt {LC}}}={1 \over R}{\sqrt {L \over C}}}
Q
=
C
R
L
C
=
R
C
L
{\displaystyle Q={CR \over {\sqrt {LC}}}={R}{\sqrt {C \over L}}}















![{\displaystyle I(t)=e^{\left(-\alpha +{\sqrt {\alpha ^{2}-\beta ^{2}}}\right)t}+e^{\left(-\alpha -{\sqrt {\alpha ^{2}-\beta ^{2}}}\right)t}=e^{-\alpha }\left[e^{\sqrt {\alpha ^{2}-\beta ^{2}}}+e^{-{\sqrt {\alpha ^{2}-\beta ^{2}}}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7f57838d46132e8865722793d4fdcfa88a25427d)



![{\displaystyle I(t)=e^{\left(-\alpha +{\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}+e^{\left(-\alpha -{\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}=e^{-\alpha }\left[e^{j\left({\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}+e^{-j\left({\sqrt {\beta ^{2}-\alpha ^{2}}}t\right)}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/40ff5495f4643e6796703fe2c08f7bc0ce6ba357)













![{\displaystyle Z_{R}+Z_{L}+Z_{C}=R+[Z_{L}-Z_{C}]=R+0=R}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6178efb58a3bb6868624d88437ba9dd10bd92c00)













