Planet Earth/1e. Earth’s Motion and Spin
Earth’s Rotation Each Day
[edit | edit source]Right now, as you are reading this, your body is traveling at an incredibly fast speed through outer space. We can calculate one component of this speed by taking Earth’s circumference based on the ellipsoid model for the Earth’s dimensions, which exhibits an equatorial circumference of 24,901.46 miles (40,075.02 km). The Earth completes a rotation around its axis every day, or more precisely every 23 hours, 56 minutes, and 4 seconds. If you are located at the equator, your velocity (speed combined with a direction) can be calculated by dividing 24,901.46 miles by 23 hours, 56 minutes, and 4 seconds, which equals 1,040.45 miles per hour. Of course, this depends on your latitude, and decreases as you approach the poles.

One way to imagine this rotation is if you have ever watched an old record album spin, or a free spinning bike wheel. The central axis of the spinning album or wheel is stationary, while the outer edges of the circle are traveling the circumference of the circle with each revolution, the further you move from the center of rotation, the quicker your speed. In other words, the larger the wheel, the faster the rotation, and the more distance is covered per unit time.

Early scientists such as Galileo, were aware of this motion and were curious as to why we do not feel this motion on the surface of the Earth. If you imagine an ant crossing a spinning record album, at the edges the ant would feel the fast motion as air zoomed by, and the pull of a centrifugal force working to fling the poor ant off the spinning record album, but as the ant crawled toward the center its feeling of motion would decrease.
The same thing can be felt if you have ever been on a merry-go-round, the closer you are toward the center the less you feel the motion of your spin. However, on Earth we do not feel like we are traveling at over a 1,000 miles per hour at the Equator, and standing still near the north or south pole.
This bizarre paradox inspired Isaac Newton to study motion, and in the process, discovered gravity, and the three laws of motion that govern how all objects move in the universe. His discoveries were published in 1687, in his book Philosophiæ Naturalis Principia Mathematica (Mathematical Principles of Natural Philosophy).
Before we can discuss why we do not feel the rotational force of the Earth, we need to define some terms.
- Speed
- is a measure of an objects’ distance traveled by the length of time the object took to travel that distance. For example, a car might have a velocity of 50 Miles per Hour (80.5 Kilometers per Hour).
- Velocity
- is speed combined with a direction in space.
- Acceleration
- is the rate of change of velocity per unit of time. For example, if a car is traveling at 50 Miles per Hour for 50 Miles and does not change speed, then it has 0 acceleration. A car that is stationary and not moving, also has 0 acceleration (within the respective frame of reference). This is because in both examples the velocity does not change.
Mathematically it is more difficult to calculate acceleration, one way to do it is to find the change of velocity for each unit of time. For example, a car going from 0 to 50 Miles Per Hour over a 5-hour long race course, we can find the speed at each 1-hour intervals and average them.
At the starting line the car is traveling at 0 miles per hour. At 1 hour the car is traveling at 10 miles per hour. At 2 hours the car is traveling at 20 miles per hour. At 3 hours the car is traveling at 30 miles per hour. At 4 hours the car is traveling at 40 miles per hour. At 5 hours the car is traveling at 50 miles per hour. Each hour the car increases its velocity by 10 miles per hour.
So, the average acceleration is equal to the average change in velocity divided by the average change in time, so the average of 10, 10, 10, 10, 10, in this example. The average acceleration is equal to 10 miles per hour, per hour (or hour squared).
If you know a little calculus, we can find what is called instantaneous acceleration, or the acceleration using the formula:
Basically, what this equation is stating is that acceleration is the derivative of velocity with respect to time.
What Isaac Newton, suggested as to the reason we do not feel this spinning motion on Earth is that the velocity of the Earth’s rotation is constant. Objects that are set into motion and have a constant velocity are said to exhibit inertia. These objects have zero acceleration.
Acceleration is when velocity changes over time. Isaac Newton realized that objects in motion will stay in motion, unless acted upon by another force. This is referred to as the law of inertia. In the weightless environment of outer space, an astronaut can spin a basketball and it will continue to spin at that velocity unless it hits another object, or another object acts against that motion. The reason that we do not feel the spin of the Earth is that everything is spinning at this constant velocity, or exhibiting the same inertia force.
However, as Isaac Newton realized, you should be feeling a centrifugal force due to this rotational force. A force is any interaction that causes an object to be moved in a direction.
Newton asked a simple question, why do objects, such as apples, fall to the Earth rather than get flung into outer space due to the rotation of the Earth?
He set about measuring the acceleration of falling objects. For example, a ball dropped from a tower. Just before the ball is dropped its velocity is 0 meters per second, but after 1 second, the ball is traveling at 10 meters per second. At 2 seconds the ball is traveling at 20 meters per second. At 3 seconds the ball is traveling at 30 meters per second. At 4 seconds the ball is traveling at 40 meters per second. At 5 seconds the ball is traveling at 50 meters per second. This sounds familiar. Each second the ball increases its velocity by 10 meters per second. So, the acceleration of the falling object is 10 meters per second per second (or second squared) or 10 m/sec2.
A century of experiments would show that falling objects on Earth’s surface have an acceleration of 9.8 m/sec2. All objects, no matter their mass, will fall at this rate.
(In reality objects will be hitting air (gas) as they fall, an object in motion will stay in motion until it is hit by another object, in this case “air” particles. This air adds resistance during free fall. So objects like parachutes, which are broad, wide and capture lots of air as they fall, or feathers will fall more slowly. Nevertheless the standard acceleration of 9.8 m/sec2 is still the same, but drag opposes this force.).
The force of a falling object is related to both mass and acceleration.
Force is measured by the mass (measured in kilograms) multiplied by the acceleration (measured in meters per second squared). Isaac Newton was rewarded by having a unit of measurement named after him!
One Newton unit of force is equal to 1-kilogram x 1 m/sec2.
Hence a bowling ball with a mass of 5 kilograms will exert a force of 5 kilograms x 9.8 m/s2 or 49 Newtons. A beach ball with a mass of 2 kilograms will exert a force of 2 kilograms x 9.8 m/s2 or 19.6 Newtons. An object measured in Newtons is a weight, since weights incorporate both mass and the acceleration. The unit of Pounds (lbs.) is also a unit of weight.
This common acceleration on the surface of the Earth is the acceleration due to gravity, which is 9.8 m/sec2. Isaac Newton realized that there was a force acting to keep objects against the surface of the Earth, and it was directly related to the mass of the Earth. The larger the mass an object had, the more its gravitational force would be. It was also related to the object’s proximity, the closer the object was, the more acceleration due to gravity the object will have. Using this mathematical relation, Newton proposed that the 9.8 m/sec2 acceleration of gravity could be used to find out how much mass the Earth had, using this formula.
g = 9.8 m/sec2 and is the acceleration of gravity on the surface of the Earth.
re = the radius of the Earth, or distance from the center of the Earth to the surface which can be found if we know the circumference of the Earth.
Me = the mass of the Earth, measured in kilograms.
G = the gravitational constant “sometimes called Big G”, a constant number, with the units of m3/kg ⋅s2.
Mass = Density x Volume. Density is the how compact a substance is and is measured relative to another substance, such as water. In other words, density is how well a substance or object floats or sinks. Volume is the cubic dimensions or space that an object occupies.
Isaac Newton did not know the value of Big G (the gravitational constant), but knew that it was a tiny number, since the mass and radius of the Earth were very large numbers, and the result of the equation had to equal 9.8 m/sec2.
The Quest to Find Big G
[edit | edit source]Newton’s work spurred a new generation of scientists trying to determine Big G, the gravitational constant. One way to determine Big G was to determine the density, volume and radius of the Earth. We can solve for Big G using this formula,
where g is the acceleration of gravity on Earth, r is the radius of the Earth from its center to the surface, D is density of Earth, and V is Earth’s volume.
One of Isaac Newton’s colleagues was Edmond Halley. Halley was one of the most brilliant scientists of the day, and is famous for his calculations of the periodicity of comets, in fact Halley’s Comet is named after him. However, he is less well known for his hypothesis that the Earth was hollow on the inside. He proposed that Earth’s density, and hence mass, was much smaller than if Earth was composed of a very dense solid inner core. During the late 1600s and early 1700s, scientists debated what the density of Earth was. Newton suggested an average density about 5 times more than water, while Halley suggested an average density less than water for the interior of the Earth. The problem was no one knew the value of Big G.
During the next century there was much discussion on the density of the Earth (the value for D). Expeditions into caverns and dark caves around the world were trying to find an entrance to the purported hollow center of the Earth. This debate captured the interest of a little short man named John Michell, who was the head of a church in Yorkshire, England, but dabbled in science in his spare time, and often wrote to fellow scientists of the day, including Benjamin Franklin. In his spare time, he thought of an experiment to measure Big G, by using a set of big very dense lead balls placed in close proximity to a set of smaller, but also very dense lead balls suspended from a string tied to a balancing rod. When the large lead balls are placed next to the smaller lead balls, the force of gravity will attract the two balls to each other. This attraction causes the balancing rod to shift slightly. To measure this movement or change in the balancing rods angle, a light was reflected off a mirror set on top of the balancing rod. Knowing the mass and radius of the lead balls, allowed one to solve for the gravitational constant, or Big G, which if known could be used to determine Earth’s density.

One of John Michell’s close friends was Henry Cavendish, a well born son of a wealthy scientist. Henry suffered from what would be called autism today, as he was incredibly shy, and struggled to carry on conversations with anyone not his close friend. Then at the age of 68, John Michell died, and left his experiment to Henry Cavendish to complete. In a large building, Henry reconstructed the experiment with the lead balls near his home, and calculated an accurate measure of Big G, the gravitational constant, which is 6.674×10−11 m3/kg⋅s2.
Using this number for Big G, it was demonstrated the Earth is not hollow, and that it is in fact, denser than rocks near the surface of the Earth which are about 3 g/cm³, with an average density of 5.51 g/cm3, or 5.5 times greater than water. This proved that the Earth is not hollow on the inside, but much denser than average rocks found on the Earth’s surface.
Henry Cavendish’s accurate calculation of the gravitational constant allows you to calculate any object’s acceleration of gravity given its mass and radius from its center of mass. The relationship between an object’s mass, radius, and the acceleration of gravity is a fundamental concept in understanding the motion of not only Earth, but other planets, moons, and stars. As well as the gravitational forces acting to hold astrological objects in orbit with each other. Furthermore, it explains why large objects in the universe take on spherical shapes around the center of mass. The acceleration of gravity also explains why we do not feel the Earth’s spin, and why objects and substances on Earth do not get flung into outer space. They are held against the Earth by its gravitational force.
Will the Earth ever stop spinning?
[edit | edit source]Should you worry about whether the Earth’s rotation or spin will slow down, and could there ever be a day in the future in when the Earth would stop rotating?
The length of the day is the time the Earth rotates once, with each longitude facing the sun once and only once during this daily rotation. If the Earth’s spin is slowing down over time, the length of the day will increase, resulting in longer days and longer nights. Today the Earth takes 23 hours, 56 minutes and 4.1 seconds to complete a rotation. (Note that it takes precisely 24 hours for the sun to reach its highest point in the sky each day, which is slightly longer than Earth’s spin, since the Earth moves a little relative to the sun each day).

Of course, the amount of daylight and night varies depending on your location and time of year, because the Earth rotates around a polar axis that is tilted at 23.5° in relationship to the sun. This is why people in Alaska (at a higher latitude) experience longer daylight during the month of July, and longer darkness during the month of December, than someone living near the Equator. The question to ask is, has the length of the Earth’s spin remained constant at 23 hours, 56 minutes and 4.1 seconds?
Like a spinning top, the Earth’s spin could be slowing down. Measuring the length of each rotation of the Earth requires clicking a very accurate stopwatch each day, and recording the time it takes for Earth to make one rotation. For the most part it says pretty close to 23 hours, 56 minutes and 4.1 seconds. However, the length does fluctuate by about 4 to 5 milliseconds. In other words, 0.004 to 0.005 seconds are added or subtracted from each day. These fluctuations appear to be on a decadal cycle, so the days in the 1860s were shorter by 0.006 seconds compared to days in the 1920s. These decadal fluctuations are believed to be the result of the transfer of angular momentum between the Earth’s fluid outer core and surrounding solid mantle, as well as tidal friction forces of the ocean as it slushes back and forth over the surface of the Earth while it spins. Weaker fluctuations occur over a yearly cycle, with days in June, July and August shorter by 0.001 seconds compared to days in December, January and February. These weaker fluctuations are cause by the atmosphere and ocean friction as the Earth spins, producing an oscillation called the “Chandler Wobble” after the American Scientist S. C. Chandler. In fact, the Earth is not just a solid mass of rock, we have a liquid ocean and a gaseous atmosphere that impacts the length of each day. It is like you are on a washing machine spinning around with wet clothes, and depending on where those clothes are in each spin cycle, there will be some variation in the speed of the spin itself.
Climate change also can have a rather important impact on the length of the day. If we were to compare the average day length during the last glacial period (25,000 years ago) to today, the day would be shorter. This is because of the Earth’s polar moment of inertia has decreased. As the great polar ice sheets that covered much of the polar regions started to melt, the distribution of the Earth’s mass shifted, from near the center of the spinning planet at the polar regions (as ice sheets), toward the equator (as melted ocean water). This change in inertia is the same phenomenon you observe when an ice skater brings his or hers arms out during a spin. The speed of the spin slows down. So as the Earth’s great ice sheets melted over the last 25,000 years, the Earth, like the spinning ice skater, projected more of its mass outward from its center toward the Equator when all that polar ice melted, slowing the spin.

While these fluctuations are interesting, they are small (several milliseconds), but we are interested in finding out when the Earth will stop spinning, and for that question we need a much longer record of day lengths, going back millions of years.
Fossil organisms keep records for the length of each year, month and day millions of years in Earth’s past. Fossil corals that live in the inter-tidal zone of the ocean are subjected to twice daily tides caused by the rotation of the Earth and gravitational pull of the moon, and amplified by the relative location of the sun. These changes in water depth result in a record in the growth rings, as well as cyclic sediments such as tidal rhythmites and banded iron formations. Using this information, Earth’s rotation has increased by 15.84 seconds every million years.
Isaac Newton proposed, objects in motion will stay in motion, unless acted upon by another force. So what force is slowing down Earth’s rotation?
The answer is our nearest neighbor— the moon!

The moon is Earth’s only natural satellite with an equatorial circumference of 10,921 km (or 6,786 miles), about 27% the size of Earth. It rotates around Earth each lunar month of 27.32 days, in an unusual orbit called a synchronous rotation. This results in the strange fact that the Moon always keeps nearly the same face or surface pointed toward Earth. The opposite side of the moon, which you do not see from Earth in the night sky, is erroneously called the “dark side” of the moon.
Both sides are illuminated once every 29.5 Earth days, as the moon rotates around Earth, resulting in different phases of illumination of the moon by the sun. The moon’s axis of rotation is only slightly tilted to 5.14° in respect to the sun, and has been slowing down by Earth’s rotation to became “tidally” locked with the Earth.
With the moon’s slower lunar month-long rotation around Earth, it acts like a slow brake applied to Earth’s spin. The Earth will slow down to match the moon’s orbit of 27.32 days or 559.68 hours. At this point the Earth will be locked with the same spin as the orbit of the moon around the Earth.
An Earth with the length of rotation equal to the current lunar month would make the days on Earth last for 27.32 days, resulting in extreme daytime and nighttime temperatures like those experienced on the life-less surface of the moon today! Is this something for you to worry about?
Not anytime soon, Earth is slowed by the braking of the Moon by just a few seconds every million years, such that it will not be until 121 billion years in the future that Earth will become locked in this death orbit with the Moon, and by then, the Earth and Moon would likely have been engulfed by an expanding Sun!
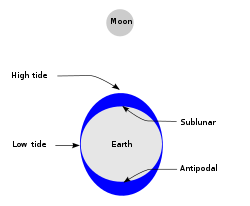
The effect of the Moon’s orbit around the Earth can be observed with shifts in the ocean tide. When the moon is positioned directly above a position on the Earth (sublunar), the ocean at that position will be pulled closer to the moon due to the moon’s gravitational force producing a high tide along the coastline. An equal high tide will be felt on the opposite or antipodal side of the Earth as well. A low tide will be observed when the moon is not located on either the sublunar or antipodal sides of the Earth. As liquid water is more directly influenced by the attraction of the Moon’s gravity than rocks that compose the solid Earth you are likely more familiar with ocean tides, but there is also Earth tides which causes the Earth to bulge with the motion of the Moon. The sun also exerts some gravitational pull on Earth and can change the magnitude of the tides depending on the seasons. You can now explain the length of a day, and the length of a lunar month, the tides, but what causes the length of a year.
Earth’s Orbit around the Sun, The Year.
[edit | edit source]
The Earth as a whole is not only spinning, but also traveling through space on an orbital path around the sun. Unlike the moon, Earth has a very dramatic tilt of its pole axis of 23.5° relative to the sun, such that during half of this voyage around the sun, the north pole faces the sun, and the south pole faces away from the sun. The tilt of Earth’s rotation of 23.5° results in longer days for the northern hemisphere when it is closer to the sun (June, July, August), and shorter days for the southern hemisphere, while the shorter days for the northern hemisphere (November, December, January) relate to longer days in the Southern Hemisphere. Because of the tilt of Earth’s axis, we have the four seasons of Summer, Fall, Winter, and Spring, which differ depending on your location in each hemisphere.
You might be surprised to learn that the orbit around the sun is not a perfect circle, as often depicted in illustrations of the solar system, but travels in an elliptical orbit around the sun. This can be demonstrated on Earth by documenting the sun’s position at noon every day of the year, which depicts a figure-8, called an analemma in the sky. The sun’s position at noon on the top of the figure 8 will happen on the day of the summer solstice, while the sun’s position at noon on the bottom of the figure 8 will happen on the day of the winter solstice, with the distance between the two points in the sky measuring Earth’s tilt of 23.5°. However, the width of the figure-8 is due to the elliptical path of the Earth around the sun. The figure-8 is not a perfect 8, but with one loop larger than the other.

This is due to the fact that Sun is not positioned directly in the center of Earth’s elliptical orbit around it. During December-January, the Earth is closer to the sun, while in June-July, the Earth is farther away. The time of year when the Earth is closest to the sun is called the Perihelion, while the time of year when the Earth is farthest from the sun is called the Aphelion.

This is opposite of what you might think, as in the Northern Hemisphere, you are closer to the sun during the cold winter months, while during the hot summer months you are farther from the sun.
The distance from the sun varies from 0.9833 AU to 1.0167 AU, where AU is the Astronomical Unit, which is the average distance between the Sun and the Earth, which is defined as 150 million kilometers (93 million miles). Hence every year the distance from the Earth to the Sun differs by about 5 million kilometers (3.1 million miles).
While Earth’s orbit around the sun may seem like a bunch of numbers and facts to memorize, the discovery that the Sun and not the Earth was the center of the solar system was a major scientific discovery. The reason for this revolution in thought was that for centuries an equally valid explanation for the yearly cycle of Earth’s orbit was proposed.
Ptolemy’s Incorrect Geocentric Model of the Solar System
[edit | edit source]
In the years shortly after the death of the Pharaoh Cleopatra and the fall of the city of Alexandria, Egypt to Roman annexation, an astronomer living in the city by the name of Claudius Ptolemy devised a model of the solar system. Ptolemy’s passion was mapping the stars, and he noticed that each night the path of Mars would move differently in reference to other stars in the night sky. Over the course of several years around 58 CE, he documented the path of Mars in the night sky demonstrating that Mars looped in the night sky over the course of several months. For example, Mars would move with the stars each night for several weeks, but then circle back for several weeks, before looping back around before heading off in the direction it started on.

Because the path of Mars looped back, Ptolemy regarded this motion as a retrograde motion, and when Mars was progressing normally with the stars, a prograde motion. Ptolemy followed the Greek tradition of Aristotle, that the Earth was the center of the universe. So why were the planets of Mars and Venus looping in the night sky, they should be traveling in straight paths across the night sky, since they were orbiting the Earth, rather than the Sun? He devised a complex geocentric model of the solar system suggesting that the orbit of Mars, as well as other known planets like Venus followed an epicycle, an additional circular orbital path in addition to their orbits around Earth. It would be a millennium and a half before Ptolemy’s model of the solar system would be disproven.
Copernicus’s Correct Heliocentric Model of the Solar System
[edit | edit source]Nicolaus Copernicus published his alternative idea in his book De revolutionibus orbium coelestium (On the Revolutions of the Heavenly Spheres) in 1543. The Heliocentric view of the Solar System placed the Sun at the center of the Solar System rather than the Earth. In doing so, Copernicus demonstrated that the epicycle orbits were actually due to the observation from Earth of the passing of Mars in its own orbit around the Sun.

Copernicus viewed the Solar System as if the planets were racing around a circular track. Earth was on the inside track, while Mars was on an outside track. As Earth moved along its track on the inside, the view of Mars on the outside track would change. The retrograde motion comes naturally as a consequence of viewing a moving Mars from the perspective of a moving Earth. Copernicus rejected the epicycles needed to produce retrograde motion, rather the planets moved in a circular orbit around the Sun. Copernicus’s book is one of the most important books in science ever published, but still needed some modification, such as the fact that the Earth rotates around the Sun in an elliptical path, as do other planets rather than a circular orbit.
How fast are you traveling through space?
[edit | edit source]At the beginning of this module we talked about how fast you are traveling through space, and used the rotation or spin of the Earth, we can now add the component of Earth’s orbit through space around the sun. The distance Earth travels around the Sun is 940 million km (584 million mi) which it accomplishes every 365.256 days. The year is not evenly divided into days so calendars have to add an extra day every 4 years, or “leap days.” We can determine the velocity of this motion around the Sun, and determine that the Earth, and everything on its surface is traveling at a remarkably fast speed around the Sun of 66,619.94 miles per hour, or 107,230.73 kilometers per hour. Imagine, if you will, that as you sit there reading this you are traveling at this incredibly fast speed on a planet sling-shooting around a Star, at 30 times the speed of the fastest airplane. Because you are!
This astonishing fact, that you are on board a fast-moving object speeding through outer space, inspired Richard Buckminster Fuller in 1969, to coin the concept that Earth is simply a spaceship traveling through the vastness of the universe. Spaceship Earth, as he called our planet, is just a giant vessel, like a battleship sailing cross an empty ocean of space. He warned that you and all life on this “spacecraft” should be prepared for a long voyage.
Earth’s Galactic Voyage
[edit | edit source]John Michell, the short fat clergyman from Yorkshire, England, who devised the experiment that proved the Earth was not hollow, proposed in a letter written in 1784 that there might be objects in the universe that have so much mass that their gravitational force of acceleration would suck in even light rays, and he called these mysterious super massive objects, dark stars. Today, we call them Black Holes. The quest to find these mysterious super massive objects in the universe was enhanced by his suggestion that gravitational effects of these objects might be seen in nearby visible bodies. However, they remained a mathematical curiosity of simply taking Newton’s equations and extrapolating them for objects with enormous mass—millions of times more than the Sun.
In 1950, no one had observed one of these super massive objects in the universe, and Jocelyn Bell, a young girl at a boarding school in England, was struggling with the female only curriculum centered around the domestic subjects of cooking and sewing. When a science class was offered only the boys were allowed to attend. Furious, she and her parents protested, and she was allowed to attend the science class with two other female students. Jocelyn Bell loved physics most of all, and in 1965 went on to study physics at the University of Cambridge. She joined a team of researchers listening for radio waves from outer space. They had been picking up blips and squawks of radio waves from faint stars. Scientists called these signals quasi-stellar radio sources, which the American astronomer Hong-Yee Chiu simplified by calling them quasars. In the summer of 1967 Jocelyn Bell and her professor Antony Hewish were looking over the printouts of the newly constructed array of radio telescopes built to detect these quasar signals from space. She noticed a regular pattern of blips every 1.3373 seconds, while tempted to attribute these radio wave patterns to aliens, they jokingly called the regular pulse of signals little green men, but realized as others had that this radio signal was produced by a super massive object with enormous gravitational forces. When viewed through a telescope, the signal was coming from a faint star, which was recognized as a Neutron Star, an extremely massive star, which was spinning at an incredibly fast rate, with a pulse of electromagnetic radiation emitted every 1.3373 seconds. These radio signals are thought to be produced as nebulous clouds of gas are pulled into these supermassive stars forming an accretion disk, which emits powerful magnetic fields and radio waves as these gases fall through the disk into the neutron star—like colossal bolts of lightning.
Scientists realized that these super massive objects could be detected by using large arrays of radio telescopes to map these signals coming from space onto the sky.
Researchers focused their attention to the center of the one of the brightest stars in the night sky, which is actually a cluster of stars called Messier 87, also known as Virgo A, the brightest point in the Virgo Constellation. It had been recognized as a cluster of stars by Charles Messier in 1781, and classified by Edwin Hubble in 1931 as an elliptical nebula of stars. Today its known as a galaxy consisting of billions of stars.
Radio waves from Messier 87 indicated that near its center is a super massive object, representing a black hole. In 2019, the Event Horizon Telescope, a network of radio telescopes, focused on this point and imaged the signals coming from its center, producing the first image of a black hole, which resembles a ghostly dark spot surrounded by light. At the center of the dark spot is an object that is 6.5 billion times the mass of the Sun and 55 million light years away.

The Event Horizon Telescope is also focused on a point in the night sky first detected by radio waves in 1974, which is thought to be the center of our own galaxy of stars, the Milky Way. In the night sky, a streak of stars appears to sweep across the night sky when viewed on an especially dark night. These stars are your closest stellar neighbors existing within your own galaxy. The Milky Way is a collection of billions of stars, including the Sun that swirl around a central point. The center of the Milky Way is located near the star Sagittarius A* (pronounced Sagittarius A-star), which is in the Sagittarius constellation. Here it has been observed that nearby stars swirl around a point, which is the location of another black hole that is 4 million times more massive than the Sun, and only 25,000 light years away. It is the nearest supermassive black hole to you.

Astronomers have measured the rate of the Sun’s rotation around this point at the center of the Milky Way Galaxy, and determined that the entire Solar System takes about 240 to 230 million years to travel around this galactic orbit around this black hole. The last time our Solar System occupied this space relative to Sagittarius A*, was before dinosaurs had evolved on Earth!
However, do not assume this passage of the Solar System around this point is slow. Earth, and the entire Solar System is zipping along this path at an incredibly fast rate of travel.
Given that 1 light year is equal 5.879 x 1012 miles, and that it takes 240 million years to travel a circumference of 157,080 million light years, or a path of 9.23471 x 1017 miles in 2.1024 x 1012 hours, our Solar System is zipping around this black hole at a velocity of 439,246 miles per hour!
You are truly on a very fast spaceship, Earth’s motion in relation to its polar axis is between 0 and 1,040.45 miles per hour (1674.44 km/hr), depending on your latitude. Earth’s motion in relation to the Sun is 66,620 miles per hour (107,214.5 km/hr), and Earth’s galactic motion in relation to Sagittarius A* is 439,246 miles per hour (706,898 km/hr).
| Previous | Current | Next | |
|---|---|---|---|
|
d. How to Navigate Across Earth using a Compass, Sextant, and Timepiece. |



