Strength of Materials/General State of Stress
Strength of Materials in Engineering Mechanics
Introduction | Introductory Concepts | Loading of Beams | Torsion | General State of Stress | Weakest link determination by use of three parameter Weibull statistics
(last) →
Principal Stresses
[edit | edit source]The general state of stress can be represented by a symmetric 3 x 3 matrix.
It is always possible to choose a coordinate system such that all shear stresses are zero. The 3 x 3 matrix is then diagonalised, with the three principal stresses on the diagonal, and all other components equal to zero. The three principal stresses are conventionally labelled σ1, σ2 and σ3.
σ1 is the maximum (most tensile) principal stress, σ3 is the minimum (most compressive) principal stress, and σ2 is the intermediate principal stress.
Stress Invariants
[edit | edit source]
Hydrostatic Stress
[edit | edit source]The so-called hydrostatic stress, σh, is given by:
Deviatoric Stresses
[edit | edit source]normal stress - pressure
where pressure = average stress
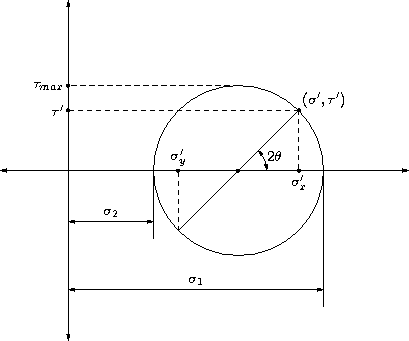
Mohr's Circle
[edit | edit source]Consider the two dimensional stress condition where the stresses are σx, σy, and τxy. We have, for another set of orthogonal axes x'-y' at angle θ with x-y, the stresses are
From the above equations, we can see that for any stress states given by σx, σy, and τxy, we can find a value of θ such that the value of σx' is maximum. This value is called the principal stress σ1 (for maximum) or σ2 (for minimum).
The principal stresses are given by
and the maximum shear stress is given by
τmax = (σ1 − σ2)/2
From the definitions for σx' and τx'y', we have
In the σ-τ graph, this is a circle with center on the x axis, and the distance of the center from the origin is given by (σx + σy)/2 and a radius given by
This circle is known as Mohr's circle, and is useful for visualizing the stress state at a point.
The above figure shows Mohr's circle for a stress state (σ, τ). The center and radius of the circle are obtained from equations stated above. The other stress σy can be read off by the point diametrically opposite the (σ, τ) point. The stress at any plane can be found using simple geometrical constructs.
Mohr's Circle for Common Cases
[edit | edit source]The above shear stress in this case is σ1/2.
A liquid is one which by definition cannot take a shear. Thus the Mohr-circle diagram is just a point. (The dot should be on the negative side of the axis as the liquids can resist only compression)
For pure shear, the Mohr circle is centered at the origin.
Failure Criteria
[edit | edit source]In the case of isotropic materials, the state of stress at any point of the body is completely defined by the triad of the principal stresses.
Now that we are able to transform stresses to get the principal stresses, we can use these stresses to consider some of the criteria (theories) postulated for the failure of materials in two- and three-axial states of stress usually based on experiments on yielding and fracture of materials in the uniaxial state of stress. According to such experiments, the kind of failure depends on the type of material. Failure of ductile materials (most of metals) occurs when the elastic limit is reached and yielding commences. Failure of non-ductile materials (e.g., cast iron, concrete) occurs by brittle fracture.
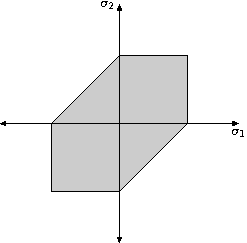
Maximum Shear Stress Criterion
[edit | edit source]For ductile materials, one failure theory is that of maximum shear stress. We know that the maximum shear stress is given by τmax = (σ1 − σ2)/2. The yield stress, σy can be determined by uniaxial tensile tests. Thus, if the maximum shear stress theory is valid, failure occurs when the maximum shear stress reaches σy/2.
In the above image, the material will fail if the stress state is outside the shaded region.
Maximum Distortion Energy Criterion
[edit | edit source]If the maximum shear stress theory is valid, failure occurs when the maximum shear stress reaches .
Failure of Materials
[edit | edit source]The actual failure mode of each material is unique, though certain criteria can be applied to classes of materials. mode of failure . fatigue failure,