Telescope Making/Project 1: The Newtonian Reflector
Project 1: The Newtonian Reflector
[edit | edit source]The Newtonian Reflector Telescope is an extremely simple and efficient design. It consists of two mirrors in a tube. That's all!

The larger, curved mirror directs incoming light to a focus. The smaller, angled mirror shunts the focused light out the side of the tube, where you can place your eye to see the image. In the Newtonian reflector, the smaller mirror is flat, but in a related design, the Cassegrain, it is convex. Read the Wikipedia article on the Newtonian Reflector for its history.
![]() Newtonian Telescope (english)
Newtonian Telescope (english)
After we consider how you will use your telescope, we will get deeply into how we choose the exact shapes and sizes of these simple pieces. Then we'll build it!
How Will You Use Your Telescope?
[edit | edit source]How will you actually use your telescope? Most folks, me included, spent a lot of time dreaming about making the telescope without giving serious thought to how we might use it. I talked about looking at stars and planets—but I also talked about looking at birds and retreating snow fields. Only when I looked through the telescope did I discover some problems. One is, the basic Newtonian telescope makes everything look upside down! Looking at the stars? This is not an issue. Looking at birds or scenery? We have a problem. Fortunately you can buy an inverting eyepiece that will turn things right side up. A second issue with the Newtonian telescope is that it is by design quite powerful. This means you might be looking at a bird's feather detail, not the bird. Third, the telescope is much larger than a pair of binoculars and needs to be supported so you can aim it and hold it steady. I don't want you to stress over this, but you need to consider, what will you do with the telescope?
Telescope Design
[edit | edit source]The telescope design covers everything from the optics (in our case the two mirrors), the brackets that hold those optics and keep them aligned, and the tube assembly that contains it all. Design also includes the supporting framework that holds the tube and lets you swivel and point it. That piece is called the telescope mount.
So let's say you think that you mainly want to look at planets and stars in the sky. Longer focal length telescopes give higher magnification. That is just how it works: the longer the focal length, the more magnified the image at your eye. Bigger mirrors will gather more light, but two telescopes with different sized mirrors will give the same size image at the focal point, if they have the same focal length (although, since more light is being put into the same size image with a larger mirror, the image in a telescope with a larger mirror will be brighter). Mirrors with a long focal length are somewhat easier to make and more forgiving of error. However, the longer the focal length, the longer the telescope, making it more difficult to move around and to store.
Shorter focal-length telescopes give less magnification, but a wider field of view. The image is a bit smaller but that is rarely an issue when looking through the eyepiece. The mirrors require a bit more work, a different technique, but they are as easy to make as the long ones. A shorter telescope is easier to move, easier to store, easier to set up for use.
There is no right answer here. The telescope you make should be one you are comfortable with and will use. I have made a lot of telescopes in the last few years. My first--I called it the Long Dog--had a truss design that took about five minutes and 10 bolts to set up. The truss poles were six feet long. It was heavy and long and you had had to stand on a ladder to reach the eyepiece, but it gave images that would take your breath away. I got one of my favorite comments while using that telescope. I showed a friend the planet Saturn. It was a perfect night, calm sky, dark, Saturn was high. He looked at the planet and said, "That is so amazing it doesn't look real." That is what we call resolution.
Regardless of the length and size of telescope you choose to make, you will find the information to build it here. I am going to do two mirrors and several mounting ideas for this, the first project of the book. I will grind and polish a shorter focal length mirror and a longer focal length mirror. Whatever you decide on I will cover it here and you will be successful with your first telescope.
Links to new terms and topics for this page:
 Newtonian Telescope (english)
Newtonian Telescope (english)
 Truss Telescope Design (english)
Truss Telescope Design (english)
The parts of the Telescope
[edit | edit source]Time to get into the details of design. The following will have some basic math and some pictures to help.
Radius of Curvature
[edit | edit source]Having considered the physical size of the telescope and the use to which I want to put it, I can now begin to consider the optics. The focal length depends on the choices made while thinking about the telescope. The focal length is set by the shape of the curve in the front of the main mirror. We make that curve by grinding the mirror. The amount of grinding and the depth of the curve are both directly affected by the choices made while thinking about the telescope.
It is the front surface of the mirror that forms the image, so it is the front surface that gets all of our attention. The back of the mirror may get quite beat up during this process. That is OK, as long as we don't crack it or put big chips in it.
The curve in the front of a telescope mirror is called the Radius of Curvature, abbreviated ROC, or sometimes just R. The front of the mirror is a smooth bowl-shaped depression. Imagine a ball, and if you have one handy, look at it while you read this. The ball has some radius, the distance from the center of the ball (an imaginary point inside where you can't see it, but it is there) to the inner surface of the ball. That is the ROC. It defines the ball's size, and the curve of the surface.
Imagine that the ball is reflective inside. Imagine that we place a source of light (a fancy way of saying put a light bulb or a flash light or something that emits light) at the center of the ball. All of the light would reflect back to the light source, at the center. However, if we move the light source away from the center, the point of reflection, or focal point, will also move away from the center in the opposite direction. When the source of light gets really far away from the center of the ball, at infinity, the focal point ends up being about half way between the center of the ball and the surface of the ball. (How far away is "infinity"? Well, figure at least 200 times the ROC.)
Now imagine that we cut off a small slice of the ball, or press the surface of the ball into some soft material, clay or moist dirt. Either way, we have a shallow, smooth, bowl-shaped depression. It would be curved just like the front surface of a telescope mirror.
The surface of the mirror has a uniform curvature that determines its focal length. The mirror is usually circular; it is a circular patch cut from that imaginary ball. It can be any size; ours will be a few inches across. (For comparison, the mirror of the Subaru telescope on Mauna Kea in Hawaii is 8.2 meters, 27 feet.) The radius of the mirror, one-half its diameter, is denoted as r.
 List of Large Telescopes (english)
List of Large Telescopes (english)
The Primary Mirror
[edit | edit source]The depth of the bowl-shaped curve that is ground into the front of the mirror is called the sagitta.
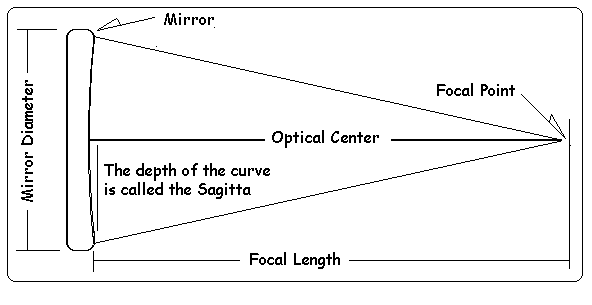
There is a math formula to figure out how deep to make the sagitta to achieve a specific focal length.
The formula relates the ROC and r, which we know to be the radius of the mirror, to S, the sagitta. The formula reads, multiply the radius of the mirror times itself, then divide it by two times the Radius of Curvature. Huh? Well, we can measure the diameter of the mirror with a ruler or tape measure and divide it in half to get the radius of the mirror. If it is eight inches across, the radius is half of that or four inches. But how much is the ROC?
Well, remember how the focal point moves as the light source moves away from the center of the ball. When the light source is at infinity, or a long way away, the focal point is halfway between the center of the ball and the surface of the ball. So the focal length is one half the Radius of Curvature, one half the ROC.
This is when we have to get specific about the telescope: what focal length did you want? Well, how long of a telescope did you want? Lets say you wanted a four-foot telescope. The ROC is twice that length, or eight feet. (If you have made a telescope before, you are fuming right now, because you know the length of the telescope has little to do with the focal length of the mirror. I'll clear it up before I'm done.)
I asked, why am I figuring out the ROC when it is just another way of saying two times the focal length? Why not just use the focal length in the Sagitta formula? Two times the ROC is equal to four times the focal length, so why not use this?
Well, you can. For our purposes as amateurs we can use either and it will be good enough.
So! let's figure out a Sagitta for an eight inch mirror with a focal length of forty eight inches.
| Sagitta calculation for a mirror eight inches in diameter and 48-inch focal length |
Not much, is it? 0.08333 inches, (and those threes go on forever). It is about the thickness of a (U.S.) penny. A ream of paper is pretty close to 2 inches thick. There are 500 sheets in a ream. 250 sheets times 0.08333 is about 21 sheets.
How accurate do you have to be? How close to a four foot telescope do you want? The focal length of the mirror will determine, to some extent, the length of the telescope tube, and for quick consideration you can assume the focal length of the mirror and the telescope tube are the same. But note that In practice the telescope tube will be as much as 25% longer than the actual focal length. If a five-foot telescope sounds too big, you can re-do the formula for a 3-foot focal length, or any other value you want.
We have spent several paragraphs on the primary mirror and I have only shared the basics. Many good books and web pages document the making of the primary mirror. Look at the links at the end of the book. Now we need to consider the second half of the optics, the secondary mirror.
The Secondary Mirror
[edit | edit source]In the diagram above (and the wikipedia page on Newtonian Telescopes) we see that the telescope gathers and focuses the light, bouncing it back up the tube. Then the optical path is turned 90 degrees and the focus and eyepiece are on the side of the tube. This is accomplished with a flat mirror set at a 45 degree angle in the tube. (It is possible in smaller telescopes to use a type of prism, but a mirror is simpler for use to make and works as well.)
 Roof Prism (english)
Roof Prism (english)
How big this secondary mirror should be, its shape, and where it should sit relative to the primary, are functions of the basic measurements of the telescope. In the previous section we used an 8 inch mirror with a 48 inch focal length as an example for our calculations. I will continue to use that example in this section.
Sizing the Tube
[edit | edit source]Before we can calculate the size and location of the secondary, we need to think about the telescope tube that supports the mirrors. How long should it be? How big in diameter? How far in will the primary mount?
The tube needs to be bigger than the primary mirror; by how much? A safe number is one inch clearance around the mirror. This works out to a tube that is two inches bigger in diameter than the primary mirror. For the 8 inch primary that is our example mirror, the tube would be 10 inches in diameter.
Tube length is determined by the focal length of the primary mirror, plus the space at the end for the primary mirror and the mirror cell—that is, the framework that supports the mirror and holds it perpendicular to the axis of the tube.
The typical primary mirror and cell form a stack about 3 to 4 inches in height. Add that to the focal length and you would have a tube that is long enough (remember, part of the focal length is turned sideways by the secondary mirror). A little extra length, say 3 or 4 more inches, gives a shield that works to block stray local light. Our example mirror has a focal length of 48 inches. Add 4 more for the mirror and cell, and 4 more for blocking light, and we have a tube 56 inches in length, and at least 10 inches in diameter.
 Vignetting (english)
Vignetting (english)
Sizing the Secondary
[edit | edit source]So, how big shall we make the secondary mirror? Before we can answer we need to consider one more key dimension, the focal offset.
A formula for figuring the size of the secondary is as follows.
In this formula,
- Os is the focal offset,
- D is the Diameter of the primary mirror
- f is focal length of the primary mirror
- d is the minor axis diameter of the secondary mirror
We multiply the diameter of the primary mirror by the focal offset and divide by the focal length of the primary mirror. This gives us a minimum minor axis. A second rule of thumb is, the secondary should be no bigger than 20 percent of the primary mirror diameter. Given an 8-inch primary, that is 1.6 inches. Purists haggle over these numbers forever. My opinion and experience are, a secondary that is a bit too big is preferable to one that is a bit too small.
Lets look at that formula in operation. I used these values:
The formula yields a size of 1.33 inches. This is a minimum size. You could increase it by 25% and have a better secondary fit. 1.33 x 1.25 = 1.66 inches—which just happens to be at the 20% mark. Pretty cool, huh?
That's it! That is how, beginning with the diameter and focal length of the primary mirror, we decide on the tube diameter and length, and the size of the secondary mirror.
The formulas are important enough that I have included them here on a graphic that will make a 3 x 5 card. Print it out!
| S = Sagitta |
| r = Radius of the primary mirror |
| f = focal length of the primary mirror |
| r = minor radius of the secondary mirror |
| Os = Offset |
| D = Diameter of the primary mirror |
| f = focal length of the primary mirror |
These formulas and the considerations that lead to them will guide your second telescope and every telescope you make after that first one. But next you need to teach your hands how to grind and polish a mirror, and that is best learned on simple inexpensive stuff.











