This Quantum World/Appendix/Calculus

Differential calculus: a very brief introduction

Another method by which we can obtain a well-defined, finite number from infinitesimal quantities is to divide one such quantity by another.

We shall assume throughout that we are dealing with well-behaved functions, which means that you can plot the graph of such a function without lifting up your pencil, and you can do the same with each of the function's derivatives. So what is a function, and what is the derivative of a function?

A function $f(x)$ is a machine with an input and an output. Insert a number $x$ and out pops the number $f(x).$ Rather confusingly, we sometimes think of $f(x)$ not as a machine that churns out numbers but as the number churned out when $x$ is inserted.

The (first) derivative $f'(x)$ of $f(x)$ is a function that tells us how much $f(x)$ increases as $x$ increases (starting from a given value of $x,$ say $x_{0}$) in the limit in which both the increase $\Delta x$ in $x$ and the corresponding increase $\Delta f=f(x+\Delta x)-f(x)$ in $f(x)$ (which of course may be negative) tend toward 0:

$f'(x_{0})=\lim _{\Delta x\rightarrow 0}{\Delta f \over \Delta x}={df \over dx}(x_{0}).$

The above diagrams illustrate this limit. The ratio $\Delta f/\Delta x$ is the slope of the straight line through the black circles (that is, the $\tan$ of the angle between the positive $x$ axis and the straight line, measured counterclockwise from the positive $x$ axis). As $\Delta x$ decreases, the black circle at $x+\Delta x$ slides along the graph of $f(x)$ towards the black circle at $x,$ and the slope of the straight line through the circles increases. In the limit $\Delta x\rightarrow 0,$ the straight line becomes a tangent on the graph of $f(x),$ touching it at $x.$ The slope of the tangent on $f(x)$ at $x_{0}$ is what we mean by the slope of $f(x)$ at $x_{0}.$

So the first derivative $f'(x)$ of $f(x)$ is the function that equals the slope of $f(x)$ for every $x.$ To differentiate a function $f$ is to obtain its first derivative $f'.$ By differentiating $f',$ we obtain the second derivative $f''={\frac {d^{2}f}{dx^{2}}}$ of $f,$ by differentiating $f''$ we obtain the third derivative $f'''={\frac {d^{3}f}{dx^{3}}},$ and so on.

It is readily shown that if $a$ is a number and $f$ and $g$ are functions of $x,$ then

${d(af) \over dx}=a{df \over dx}$  and  ${d(f+g) \over dx}={df \over dx}+{dg \over dx}.$

A slightly more difficult problem is to differentiate the product $e=fg$ of two functions of $x.$ Think of $f$ and $g$ as the vertical and horizontal sides of a rectangle of area $e.$ As $x$ increases by $\Delta x,$ the product $fg$ increases by the sum of the areas of the three white rectangles in this diagram:

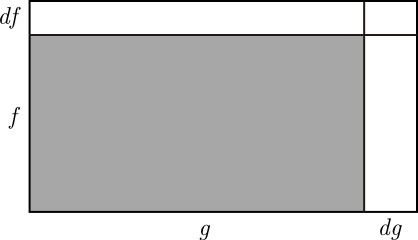
In other "words",

$\Delta e=f(\Delta g)+(\Delta f)g+(\Delta f)(\Delta g)$

and thus

${\frac {\Delta e}{\Delta x}}=f\,{\frac {\Delta g}{\Delta x}}+{\frac {\Delta f}{\Delta x}}\,g+{\frac {\Delta f\,\Delta g}{\Delta x}}.$

If we now take the limit in which $\Delta x$ and, hence, $\Delta f$ and $\Delta g$ tend toward 0, the first two terms on the right-hand side tend toward $fg'+f'g.$ What about the third term? Because it is the product of an expression (either $\Delta f$ or $\Delta g$) that tends toward 0 and an expression (either $\Delta g/\Delta x$ or $\Delta f/\Delta x$) that tends toward a finite number, it tends toward 0. The bottom line:

$e'=(fg)'=fg'+f'g.$

This is readily generalized to products of $n$ functions. Here is a special case:

$(f^{n})'=f^{n-1}\,f'+f^{n-2}\,f'\,f+f^{n-3}\,f'\,f^{2}+\cdots +f'\,f^{n-1}=n\,f^{n-1}f'.$

Observe that there are $n$ equal terms between the two equal signs. If the function $f$ returns whatever you insert, this boils down to

$(x^{n})'=n\,x^{n-1}.$

Now suppose that $g$ is a function of $f$ and $f$ is a function of $x.$ An increase in $x$ by $\Delta x$ causes an increase in $f$ by $\Delta f\approx {\frac {df}{dx}}\Delta x,$ and this in turn causes an increase in $g$ by $\Delta g\approx {\frac {dg}{df}}\Delta f.$ Thus ${\frac {\Delta g}{\Delta x}}\approx {\frac {dg}{df}}{\frac {df}{dx}}.$ In the limit $\Delta x\rightarrow 0$ the $\approx$ becomes a $=$ :

${dg \over dx}={dg \over df}{df \over dx}.$

---

We obtained $(x^{n})'=n\,x^{n-1}$ for integers $n\geq 2.$ Obviously it also holds for $n=0$ and $n=1.$

1. Show that it also holds for negative integers $n.$ Hint: Use the product rule to calculate $(x^{n}x^{-n})'.$
2. Show that $({\sqrt {x}})'=1/(2{\sqrt {x}}).$ Hint: Use the product rule to calculate $({\sqrt {x}}{\sqrt {x}})'.$
3. Show that $(x^{n})'=n\,x^{n-1}$ also holds for $n=1/m$ where $m$ is a natural number.
4. Show that this equation also holds if $n$ is a rational number. Use ${dg \over dx}={dg \over df}{df \over dx}.$

Since every real number is the limit of a sequence of rational numbers, we may now confidently proceed on the assumption that $(x^{n})'=n\,x^{n-1}$ holds for all real numbers $n.$