Topology/Connectedness
Motivation
[edit | edit source]To best describe what is a connected space, we shall describe first what is a disconnected space. A disconnected space is a space that can be separated into two disjoint groups, or more formally:
A space is said to be disconnected iff a pair of disjoint, non-empty open subsets exists, such that .
A space that is not disconnected is said to be a connected space.
Examples
[edit | edit source]- A closed interval is connected. To show this, suppose that it was disconnected. Then there are two nonempty disjoint open sets and whose union is . Let be the set equal to or and which does not contain . Let . Since X does not contain b, s must be within the interval [a,b] and thus must be within either X or . If is within , then there is an open set within . If is not within , then is within , which is also open, and there is an open set within . Either case implies that is not the supremum.
- The topological space is disconnected:
A picture to illustrate: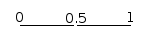
As you can see, the definition of a connected space is quite intuitive; when the space cannot be separated into (at least) two distinct subspaces.
Definitions
[edit | edit source]Definition 1.1
A subset of a topological space is said to be clopen if it is both closed and open.
Definition 1.2
A topological space X is said to be totally disconnected if every subset of X having more than one point is disconnected under the subspace topology
Theorems about connectedness
[edit | edit source]If and are homeomorphic spaces and if is connected, then is also connected.
Proof:
Let be connected, and let be a homeomorphism. Assume that is disconnected. Then there exists two nonempty disjoint open sets and whose union is . As is continuous, and are open. As is surjective, they are nonempty and they are disjoint since and are disjoint. Moreover, , contradicting the fact that is connected. Thus, is connected.
Note: this shows that connectedness is a topological property.
If two connected sets have a nonempty intersection, then their union is connected.
Proof:
Let and be two non-disjoint, connected sets. Let and be non-empty open sets such that . Let .
Without loss of generality, assume .
As is connected, ...(1).
As is non-empty, such that .
Hence, similarly, ...(2)
Now, consider . From (1) and (2), , and hence . As are arbitrary, is connected.
If two topological spaces are connected, then their product space is also connected.
Proof:
Let X1 and X2 be two connected spaces. Suppose that there are two nonempty open disjoint sets A and B whose union is X1×X2. If for every x∈X, {x}×X2 is either completely within A or within B, then π1(A) and π1(B) are also open, and are thus disjoint and nonempty, whose union is X1, contradicting the fact that X1 is connected. Thus, there is an x∈X such that {x}×X2 contains elements of both A and B. Then π2(A∩{(x,y)}) and π2(B∩{(x,y)}), where y is any element of X2, are nonempty disjoint sets whose union is X2, and which are a union of open sets in {(x,y)} (by the definition of product topology), and are thus open. This implies that X2 is disconnected, a contradiction. Thus, X1×X2 is connected.
Exercises
[edit | edit source]- Show that a topological space is disconnected if and only if it has clopen sets other than and (Hint: Why is clopen?)
- Prove that if is continuous and surjective (not necessarily homeomorphic), and if is connected, then is connected.
- Prove the Intermediate Value Theorem: if is continuous, then for any between and , there exists a such that .
- Prove that is not homeomorphic to (hint: removing a single point from makes it disconnected).
- Prove that an uncountable set given the countable complement topology is connected (this space is what mathematicians call 'hyperconnected')
- a)Prove that the discrete topology on a set X is totally disconnected.
b) Does the converse of a) hold (Hint: Even if the subspace topology on a subset of X is the discrete topology, this need not imply that the set has the discrete topology)





![{\displaystyle [a,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9c4b788fc5c637e26ee98b45f89a5c08c85f7935)




![{\displaystyle [a,b]\setminus X}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e887507a0b2538b7fcc66a463818525854347931)




























![{\displaystyle f:[a,b]\to \mathbb {R} }](https://wikimedia.org/api/rest_v1/media/math/render/svg/b592d102ccd1ba134d401c5b3ea177baaba3ffac)



![{\displaystyle c\in [a,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/997256364b06acf0710e5d24da39e8c42991a249)


