Traditional Abacus and Bead Arithmetic/Division/Modern and traditional division; close relatives
Introduction
[edit | edit source]As explained in the previous chapter, there are two main methods of division used with the abacus: the modern division and the traditional division. The modern method of division (MD), shojohou in Japanese, shāng chúfǎ in Chinese (商除法) , is actually the oldest, dating back to approx. 200 CE and only makes use of the multiplication table. By comparison, the traditional method (TD), kijohou, guī chúfǎ (帰除法), is more recent but also very old, dating back to the times of counting rods, at least from the 13th century. This method makes use of both the multiplication table and a specific division table. TD has been the standard method studied with the abacus for at least 4 centuries[1][2], losing popularity in the 1930s. The reason for this is that modern abacus students already know how to divide with pencil and paper before embarking on the study of the abacus and, having a very tight study program or being very busy, it is not a question of spending time learning a new method of division and memorizing a new table, but of taking advantage of what is already known; MD is the closest thing to written long division that can be done on the abacus.
It would be difficult to say which of the two division methods is more efficient, Kojima[3] does not dare to say it, what does seem generally accepted is that the traditional method is more comfortable or relaxed since one does not have to think about anything, just follow the rules. From what follows, one can think that MD is somewhat more efficient (faster) than TD, one could say that while MD is for the sprinter, TD is for the Marathon runner; i.e. for those who have to spend many hours a day doing divisions…
At first glance it may seem that these two methods of division are very different from each other, we will show in what follows that the two methods are so similar and related that they can be considered close relatives (with MD being the older brother and TD the youngest) to the point that if you are already skilled in MD you are also skilled in TD! … although you don't know it yet and you are still far from getting all the power of TD.
For this purpose, we will go back to older division methods in order to position MD and TD within the framework of the Chunking methods[4] (sometimes also called the partial quotients method or the hangman method) which will allow us to show the extreme similarity of both approaches. After this, we will delve into the hidden beauty of TD and understand why it simplifies life with the abacus. In what follows we assume that you already know all about the modern division with the abacus, how to revise up and down, etc. as explained, for instance, by Kojima.
First methods
[edit | edit source]Let's take 1225÷35 = 35 as an example. There is no simpler way to proceed than by repeated subtraction and since 35 is greater than the first two digits of the dividend, we will start subtracting 35 from 122 using a column from the abacus as a counter.
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | Start, counter in D, |
| 35 1 875 | subtract 35 from GH, add 1 to counter D, |
| 35 2 525 | subtract 35 from GH, add 1 to counter D, |
| 35 3 175 | subtract 35 from GH, add 1 to counter D, |
| 35 31 140 | subtract 35 from HI, add 1 to counter E, |
| 35 32 105 | subtract 35 from HI, add 1 to counter E, |
| 35 33 70 | subtract 35 from HI, add 1 to counter E, |
| 35 34 35 | subtract 35 from HI, add 1 to counter E, |
| 35 35 00 | subtract 35 from HI, add 1 to counter E. |
| 35 35 | No remainder. Done, quotient is 35! |
That was easy but a little long. If we can easily double the divisor and retain it in memory, we can shorten the operation by subtracting one or two times the divisor chunks.
| times | chunks |
|---|---|
| 1 | 35 |
| 2 | 70 |
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | Start, counter in D, |
| 35 2 525 | subtract 70 from GH, add 2 to counter D, |
| 35 3 175 | subtract 35 from GH, add 1 to counter D, |
| 35 32 105 | subtract 70 from HI, add 2 to counter E, |
| 35 34 35 | subtract 70 from HI, add 2 to counter E, |
| 35 35 00 | subtract 35 from HI, add 1 to counter E. |
| 35 35 | No remainder. Done, quotient is 35! |
Or even better if we can build a table like the one below by doubling the divisor three times[5]:
| times | chunks |
|---|---|
| 1 | 35 |
| 2 | 70 |
| 4 | 140 |
| 8 | 280 |
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | Start, counter in D, |
| 35 2 525 | subtract 70 from GH, add 2 to counter D, |
| 35 3 175 | subtract 35 from GH, add 1 to counter D, |
| 35 34 35 | subtract 140 from HI, add 4 to counter E, |
| 35 35 0 | subtract 35 from HI, add 1 to counter E. |
| 35 35 | No remainder. Done, quotient is 35! |
which is somewhat shorter and, clearly, nothing could be faster than having a complete multiplication table of the divisor
| times | chunks |
|---|---|
| 1 | 35 |
| 2 | 70 |
| 3 | 105 |
| 4 | 140 |
| 5 | 175 |
| 6 | 210 |
| 7 | 245 |
| 8 | 280 |
| 9 | 315 |
then
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | Start, counter in D, |
| 35 3 175 | subtract 105 from GH, add 3 to counter D, |
| 35 35 00 | subtract 175 from HI, add 5 to counter E. |
| 35 35 | No remainder. Done, quotient is 35! |
There is no doubt, this is an optimal division method, nothing can be faster and more comfortable ... once we have a chunk table like the one above. But calculating the chunk table is time consuming and requires paper and pencil to write it and this extra work would only be justified if we have a large number of divisions to do with the same common divisor.
In 1617 John Napier, the father of logarithms, presented his invention to alleviate this problem consisting of a series of rods, known as Napier's Bones, with the one-digit multiplication table written on them and that could be combined to get the multiplication table of any number. For example, in our case

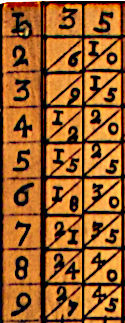
|
1 | 35 |
| 2 | 70 | |
| 3 | 105 | |
| 4 | 140 | |
| 5 | 175 | |
| 6 | 210 | |
| 7 | 245 | |
| 8 | 280 | |
| 9 | 315 |
There is no doubt that such an invention spread to the East and was used in conjunction with the abacus, but this use must be considered as exceptional; not everyone had Napier bones close at hand. Another tool is needed and that tool is the multiplication table learned by heart.
It should be noted that the above procedures do not exhaust the possibilities of the chunking methods. If you read The Definitive Higher Math Guide on Integer Long Division[4] article, you will be amazed at the variety of division methods that can be performed. Both MD and TD used in the abacus belong to this category, as we are going to see.
Modern Division (商除法)
[edit | edit source]One of the key points of learning abacus is to be aware that this instrument allows us to correct some things very quickly and without leaving traces and this is specially useful in the case of division. So if we have to divide 634263÷79283, instead of busting our brain trying to find the correct quotient figure, we simply choose an approximate provisional or interim figure by simplifying the original problem to 63÷7 and test it by trying to subtract the chunk (interim quotient digit)✕79283 from the dividend; one of the following will occur:
- The interim quotient digit is correct
- It is excessive and we must revise it down
- It is insufficient and we must revise it up
Let's see it applied to our previous example. Instead of directly trying to solve 1225÷35 we simplify and try to solve 12÷3 using the memorized multiplication by 3 table.
| 3×1 | 3 |
| 3×2 | 6 |
| 3×3 | 9 |
| 3×4 | 12 |
| 3×5 | 15 |
| 3×6 | 18 |
| 3×7 | 21 |
| 3×8 | 24 |
| 3×9 | 27 |
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | 12÷3↦4 from the table above as 3×4=12 |
| +4 | enter interim quotient in E |
| 35 41225 | Now try to subtract the chunk 4✕35 from FGH, |
| -12 | first 4✕3 from FG |
| 35 40025 | then 4✕5 from GH |
| -20 | Cannot subtract! |
| -1 | Revising down interim quotient digit |
| 35 30025 | |
| +3 | return the excess subtracted from FG |
| 35 30325 | |
| -15 | continue normally, subtract 3✕5 from GH |
| 35 3 175 | 17÷3↦5 from the table above as 3×5=15 |
| +5 | enter interim quotient in F |
| 35 35175 | Try to subtract chunk 5✕35 from GHI |
| -15 | first 5✕3 from GH |
| 35 35025 | |
| -25 | then 5✕5 from HI |
| 35 35 | No remainder, done! 1225÷35 = 35 |
Traditional Division (帰除法)
[edit | edit source]Instead of directly trying to solve the original problem 1225÷35 or the approximation used in MD 12÷3, we simplify still more and try to solve 10÷3; that is, we use a cruder approach to the original problem by ignoring the second digit of the dividend, so we must prepare to revise the interim quotient more frequently. By this change of focus from 12÷3 to 10÷3 we are adopting the philosophy of TD; it is only a slight variation of the chunking technique used in MD. This is why we can consider both division mechanisms as close relatives, members of the chunking methods family of division algorithms… and this is also why it can be said that if you are already proficient in modern division you are also already proficient in traditional division! but let us follow...
Continuing with our example
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | 10÷3↦3 from multiplication table |
| +3 | enter interim quotient in E |
| 35 31225 | Try to subtract chunk 3✕35 from FGH, |
| -09 | first 3✕3 from FG |
| 35 3 325 | |
| -15 | then 3✕5 from GH |
| 35 3 175 | ok. |
| 35 3 175 | 10÷3↦3 |
| +3 | enter interim quotient in F |
| 35 33175 | Try to subtract 3✕35 from GHI, |
| -09 | first 3✕3 from GH |
| 35 33 85 | |
| -15 | then 3✕5 from HI |
| 35 33 70 | remainder greater than divisor (35) |
| +1-35 | Revising up |
| 35 34 35 | remainder equal to divisor (35) |
| +1-35 | Revising up again |
| 35 35 | No remainder, done! 1225÷35 = 35 |
Note that MD and TD, as explained so far, can be freely intermixed during the same division problem. This is an interesting and recommended exercise that allows you to compare both strategies side by side.
TS uses a simpler and lower approach to the original problem than MD, so that we can foresee some pros and cons
- Pros
- Some may consider this approach simpler
- It will be necessary to revise down less frequently (revising down is usually more difficult and prone to mistakes than revising up)
- Cons
- We need to revise the interim quotient more frequently, which is an efficiency issue.
The previous two pros probably played a role in the development of the sophisticated technique we know as traditional division, but understanding why it was the preferred method for centuries, despite the above con, requires reflecting on the origin of the mental effort made during division and discovering the hidden beauty of TD.
The source of mental effort
[edit | edit source]When we learn the multiplication table we memorize a sequence of phrases like:
- “nine times nine , eighty-one”
- “nine times eight, seventy-two”
- ...
The order in which these phrases are learned can vary, but the structure of the phrases is similar in all languages, at least it is in Chinese and Japanese. It consists of a label that contains the two factors to be multiplied followed by the product. As soon as we think of the label, it, acting as an invocation, calls to our consciousness the value of the product. Let us represent it in the following way (read ➡ as the invocation):
| Language | Label | Product | |
|---|---|---|---|
| English | nine times nine | ➡ | eighty-one |
| Chinese | 九九 | ➡ | 八十一 |
| Japanese | くく | ➡ | はちじゅういち |
| Symbolic | 9✕9 | ➡ | 81 |
How do we use this multiplication table during division? Let's think about our example above using shojohou or modern division method: 17÷3↦5, from the multiplication by three table we need the largest product that can be subtracted from 17. We need to scan in our memory (represented by ⤷) at least a few lines of said table and for each product rescued, see if it is less than 17 and choose the maximum of those less than 17. A complicated process that can be represented as:
| 3✕1 | ➡ | 3 | |||
| 3✕2 | ➡ | 6 | |||
| ⤷ | 3✕3 | ➡ | 9 | yes | |
| ⤷ | 3✕4 | ➡ | 12 | yes | |
| ⤷ | 3✕5 | ➡ | 15 | yes | select this one! |
| ⤷ | 3✕6 | ➡ | 18 | no | |
| 3✕7 | ➡ | 21 | |||
| 3✕8 | ➡ | 24 | |||
| 3✕9 | ➡ | 27 |
This process is time and energy consuming. Computer specialists might find a similarity between this process and searching a relational database table on a non-indexed column; the inefficiency of such a search is well known. Creating a new index or key for that table based on the column and the search criteria can improve things drastically. Can we do something similar in our case to make the division more comfortable?
Indexing the multiplication table (division table)
[edit | edit source]To do something similar to indexing the multiplication table in terms of the products to facilitate the search, we should memorize new phrases that contain those products as labels; that is, phrases that begin with them; for instance:
| Label | Quotient |
|---|---|
| 3/3 | 1 |
| 6/3 | 2 |
| 9/3 | 3 |
| 12/3 | 4 |
| 15/3 | 5 |
| 18/3 | 6 |
| 21/3 | 7 |
| 24/3 | 8 |
| 27/3 | 9 |
That is, we have to memorize a division table, which is a hard work. Also think that the table above is not optimal in the sense that much of the numbers between 1 and 29 are missing; perhaps we should memorize a table of the following style instead:
| Label | Quotient | Remainder |
|---|---|---|
| 1/3 | 0 | 1 |
| 2/3 | 0 | 2 |
| 3/3 | 1 | 0 |
| 4/3 | 1 | 1 |
| 5/3 | 1 | 2 |
| … | … | … |
| 27/3 | 9 | 0 |
| 28/3 | 9 | 1 |
| 29/3 | 9 | 2 |
where the third column contains the remainders of the euclidean division. You will probably agree that memorizing such a table is out of the reach of ordinary humans (think of the table for 9!).
The hidden beauty of traditional division
[edit | edit source]If we dedicate a lifetime to dividing with the abacus using MD method we would end up facing all elementary divisions of the type ab÷c where a, b and c are digits and ab < c0, about 360 in total. However, if we were to use TD, we would be faced with all elemental divisions of the type a0÷c or (10✕a)÷c, only 36 in total! ... and this makes the memorization of a division table viable. In fact, to divide by 3 it is enough to memorize:
| Label | Quotient | Remainder |
|---|---|---|
| 10/3 | 3 | 1 |
| 20/3 | 6 | 2 |
or, in a more compact symbolic form
| Rule |
|---|
| 1/3 > 3+1 |
| 2/3 > 6+2 |
that we can use directly to solve our example without any thinking by simply choosing the figure suggested by the rule as the interim quotient.
| Abacus | Comment |
|---|---|
| ABCDEFGHI | |
| 35 1225 | Use rule 1/3 > 3+1 |
| +3 | enter interim quotient in E |
| 35 31225 | Try to subtract chunk 3✕35 from FGH, |
| -09 | first 3✕3 from FG |
| 35 3 325 | |
| -15 | then 3✕5 from GH |
| 35 3 175 | ok. |
| 35 3 175 | Use rule 1/3 > 3+1 |
| +3 | enter interim quotient in F |
| 35 33175 | Try to subtract 3✕35 from GHI, |
| -09 | first 3✕3 from GH |
| 35 33 85 | |
| -15 | then 3✕5 from HI |
| 35 33 70 | remainder greater than divisor (35) |
| +1-35 | Revising up |
| 35 34 35 | remainder equal to divisor (35) |
| +1-35 | Revising up again |
| 35 35 | No remainder, done! 1225÷35 = 35 |
but we have not yet made use of the remainder that appears in the rules after the plus sign; that and other issues will be covered in the next chapter.
The division table
[edit | edit source]Let's conclude by offering the complete division table used in TD. All elements are obtained from a0÷c terms by euclidean division.
| 1/9>1+1 | 2/9>2+2 | 3/9>3+3 | 4/9>4+4 | 5/9>5+5 | 6/9>6+6 | 7/9>7+7 | 8/9>8+8 | 9/9>9+9 |
| 1/8>1+2 | 2/8>2+4 | 3/8>3+6 | 4/8>5+0 | 5/8>6+2 | 6/8>7+4 | 7/8>8+6 | 8/8>9+8 | |
| 1/7>1+3 | 2/7>2+6 | 3/7>4+2 | 4/7>5+5 | 5/7>7+1 | 6/7>8+4 | 7/7>9+7 | ||
| 1/6>1+4 | 2/6>3+2 | 3/6>5+0 | 4/6>6+4 | 5/6>8+2 | 6/6>9+6 | |||
| 1/5>2+0 | 2/5>4+0 | 3/5>6+0 | 4/5>8+0 | 5/5>9+5 | ||||
| 1/4>2+2 | 2/4>5+0 | 3/4>7+2 | 4/4>9+4 | |||||
| 1/3>3+1 | 2/3>6+2 | 3/3>9+3 | ||||||
| 1/2>5+0 | 2/2>9+2 | |||||||
| 1/1>9+1 |
References
[edit | edit source]- ↑ Xú Xīnlǔ (徐心魯) (1993) [1573]. Pánzhū Suànfǎ (盤珠算法) (in Chinese). Zhōngguó kēxué jìshù diǎnjí tōng huì (中國科學技術典籍通彙).
{{cite book}}: Unknown parameter|trans_title=ignored (|trans-title=suggested) (help) - ↑ Shinoda, Shosaku (篠田正作) (1895). Jitsuyo Sanjutsu (実用算術) (in Japanese).
{{cite book}}: Unknown parameter|trans_title=ignored (|trans-title=suggested) (help) - ↑ Kojima, Takashi (1954), The Japanese Abacus: its Use and Theory, Tokyo: Charles E. Tuttle Co., Inc., ISBN 978-0-8048-0278-9
- ↑ a b "The Definitive Higher Math Guide on Integer Long Division (and Its Variants)". Math Vault. Archived from the original on May 14, 2021.
{{cite web}}: Unknown parameter|accesdate=ignored (|access-date=suggested) (help); Unknown parameter|name=ignored (help) - ↑ Wilson, Jeff. "Long Division Teaching Aid, "Double Division"". Double Division. Archived from the original on March 02, 2021.
{{cite web}}: Check date values in:|archivedate=(help); Text "year 2005" ignored (help); Unknown parameter|accesdate=ignored (|access-date=suggested) (help)
Further readings
[edit | edit source]- "The Definitive Higher Math Guide on Integer Long Division (and Its Variants)". Math Vault. Archived from the original on May 14, 2021.
{{cite web}}: Unknown parameter|accesdate=ignored (|access-date=suggested) (help) - *Knott, Cargill G. (1886), "The Abacus, in its Historic and Scientific Aspects", Transactions of the Asiatic Society of Japan, 14: 18–73 deals with traditional division
- Totton Heffelfinger (2013). "Suan Pan and the Unit Rod - Division". 算盤 Abacus: Mystery of the Bead. Archived from the original on August 3, 2021.
{{cite web}}: Unknown parameter|accesdate=ignored (|access-date=suggested) (help) - Totton Heffelfinger (2013). "Short Division Techniques - Chinese Suan Pan". 算盤 Abacus: Mystery of the Bead. Archived from the original on August 3, 2021.
{{cite web}}: Unknown parameter|accesdate=ignored (|access-date=suggested) (help) - Totton Heffelfinger (2013). "Long Division Techniques - Chinese Suan Pan". 算盤 Abacus: Mystery of the Bead. Archived from the original on August 3, 2021.
{{cite web}}: Unknown parameter|accesdate=ignored (|access-date=suggested) (help) - Totton Heffelfinger (2013). "Chinese Division Rules on a Soroban". 算盤 Abacus: Mystery of the Bead. Archived from the original on August 3, 2021.
{{cite web}}: Unknown parameter|accesdate=ignored (|access-date=suggested) (help)
