Waves/2-3D Problems

Waves : 2 and 3 Dimension Waves
1 - 2 - 3 - 4 - 5 - 6 - 7
Problems

Problems

Point A is at the origin. Point B is $3{\mbox{ m}}$ $3{\mbox{ m}}$ distant from A at $30^{\circ }$ $30^{\circ }$ counterclockwise from the $x$ $x$ axis. Point C is $2{\mbox{ m}}$ $2{\mbox{ m}}$ from point A at $100^{\circ }$ $100^{\circ }$ counterclockwise from the $x$ $x$ axis.
1. Obtain the Cartesian components of the vector ${\mbox{D}}_{1}$ which goes from A to B and the vector ${\mbox{D}}_{2}$ which goes from A to C.
2. Find the Cartesian components of the vector ${\mbox{D}}_{3}$ which goes from B to C.
3. Find the direction and magnitude of ${\mbox{D}}_{3}$ .
For the vectors in the previous problem, find ${\mbox{D}}_{1}\cdot {\mbox{D}}_{2}$ using both the cosine form of the dot product and the Cartesian form. Check to see if the two answers are the same.
Show graphically or otherwise that $\vert {\mbox{A}}+{\mbox{B}}\vert \neq \vert {\mbox{A}}\vert +\vert {\mbox{B}}\vert$ except when the vectors ${\mbox{A}}$ and ${\mbox{B}}$ are parallel.
A wave in the $x$ $x$ - $y$ $y$ plane is defined by $h=h_{0}\sin({\mbox{k}}\cdot {\mbox{x}})$ $h=h_{0}\sin({\mbox{k}}\cdot {\mbox{x}})$ where ${\mbox{k}}=(1,2){\mbox{ cm}}^{-1}$ ${\mbox{k}}=(1,2){\mbox{ cm}}^{-1}$ .
1. On a piece of graph paper draw $x$ and $y$ axes and then plot a line passing through the origin which is parallel to the vector ${\mbox{k}}$ .
2. On the same graph plot the line defined by ${\mbox{k}}\cdot {\mbox{x}}=k_{x}x+k_{y}y=0$ , ${\mbox{k}}\cdot {\mbox{x}}=\pi$ , and ${\mbox{k}}\cdot {\mbox{x}}=2\pi$ . Check to see if these lines are perpendicular to ${\mbox{k}}$ .
Two deep plane ocean waves with the same frequency $\omega$ $\omega$ are moving approximately to the east. However, one wave is oriented a small angle $\beta$ $\beta$ north of east and the other is oriented $\beta$ $\beta$ south of east.
1. Determine the orientation of lines of constructive interference between these two waves.
2. Determine the spacing between lines of constructive interference.
An example of waves with a dispersion relation in which the frequency is a function of both wave vector magnitude and direction is shown graphically in figure 2.20.
1. What is the phase speed of the waves for each of the three wave vectors? Hint: You may wish to obtain the length of each wave vector graphically.
2. For each of the wave vectors, what is the orientation of the wave fronts?
3. For each of the illustrated wave vectors, sketch two other wave vectors whose average value is the illustrated vector, and whose tips lie on the same frequency contour line. Determine the orientation of lines of constructive interference produced by the superimposing pairs of plane waves for which each of the vector pairs are the wave vectors.

Figure 2.20: Graphical representation of the dispersion relation for shallow water waves in a river flowing in the

x

direction. Units of frequency are hertz, units of wavenumber are inverse meters.

Two gravity waves have the same frequency, but slightly different wavelengths.
1. If one wave has an orientation angle $\theta =\pi /4{\mbox{ radians}}$ , what is the orientation angle of the other? (See figure 2.6.)
2. Determine the orientation of lines of constructive interference between these two waves.
A plane wave impinges on a single slit, spreading out a half-angle $\alpha$ after the slit. If the whole apparatus is submerged in a liquid with index of refraction $n=1.5$ , how does the spreading angle of the light change? (Hint: Recall that the index of refraction in a transparent medium is the ratio of the speed of light in a vacuum to the speed in the medium. Furthermore, when light goes from a vacuum to a transparent medium, the light frequency doesn't change. Therefore, how does the wavelength of the light change?)
Determine the diameter of the telescope needed to resolve a planet $2\times 10^{8}{\mbox{ km}}$ from a star which is $6{\mbox{ light years}}$ from the earth. (Assume blue light which has a wavelength $\lambda \approx 4\times 10^{-7}{\mbox{ m}}=400{\mbox{ nm}}$ . Also, don't worry about the great difference in brightness between the two for the purposes of this problem.)
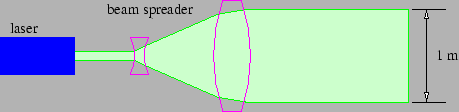
A laser beam from a laser on the earth is bounced back to the earth by a corner reflector on the moon.
1. Engineers find that the returned signal is stronger if the laser beam is initially spread out by the beam expander shown in figure 2.21. Explain why this is so.
2. The beam has a diameter of $1{\mbox{ m}}$ leaving the earth. How broad is it when it reaches the moon, which is $4\times 10^{5}{\mbox{ km}}$ away? Assume the wavelength of the light to be $5\times 10^{-7}{\mbox{ m}}$ .
3. How broad would the laser beam be at the moon if it weren't initially passed through the beam expander? Assume its initial diameter to be $1{\mbox{ cm}}$ .

Figure 2.21: Sketch of a beam expander for a laser.

Suppose that a plane wave hits two slits in a barrier at an angle, such that the phase of the wave at one slit lags the phase at the other slit by half a wavelength. How does the resulting interference pattern change from the case in which there is no lag?
Suppose that a thin piece of glass of index of refraction $n=1.33$ $n=1.33$ is placed in front of one slit of a two slit diffraction setup.
1. How thick does the glass have to be to slow down the incoming wave so that it lags the wave going through the other slit by a phase difference of $\pi$ ? Take the wavelength of the light to be $\lambda =6\times 10^{-7}{\mbox{ m}}$ .
2. For the above situation, describe qualitatively how the diffraction pattern changes from the case in which there is no glass in front of one of the slits. Explain your results.
A light source produces two wavelengths, $\lambda _{1}=400{\mbox{ nm}}$ $\lambda _{1}=400{\mbox{ nm}}$ (blue) and $\lambda _{2}=600{\mbox{ nm}}$ $\lambda _{2}=600{\mbox{ nm}}$ (red).
1. Qualitatively sketch the two slit diffraction pattern from this source. Sketch the pattern for each wavelength separately.
2. Qualitatively sketch the 16 slit diffraction pattern from this source, where the slit spacing is the same as in the two slit case.
A light source produces two wavelengths, $\lambda _{1}=631{\mbox{ nm}}$ and $\lambda _{2}=635{\mbox{ nm}}$ . What is the minimum number of slits needed in a grating spectrometer to resolve the two wavelengths? (Assume that you are looking at the first order diffraction peak.) Sketch the diffraction peak from each wavelength and indicate how narrow the peaks must be to resolve them.